A Free Boundary Problem for Thin Films
Presenter
March 8, 2011
Keywords:
- applied PDE
- partial differential equations
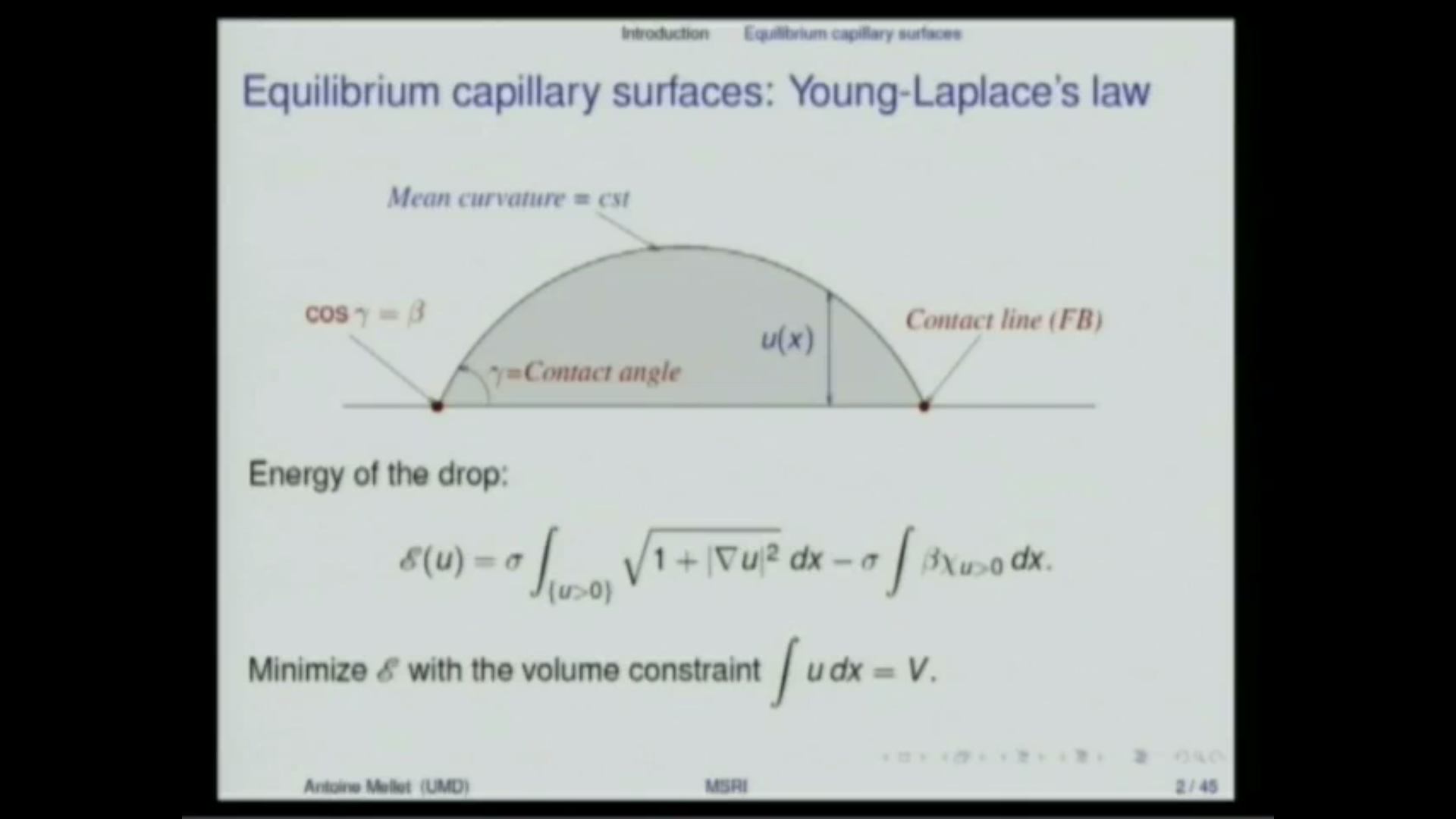
- thin film equation
- boundary layers and boundary conditions
- regularization
- contact angle
- capillary forces
MSC:
- 35R35
- 35R37
- 35-xx
- 35Qxx
- 76D27
- 76D45
- 74K35
- 74Kxx
- 35Q35
Abstract
The lubrication approximation leads to a fourth order degenerate equation modelling the evolution of small viscous droplets on a solid support (the thin film equation). Along the free boundary (contact line), the solution must satisfy a gradient condition (contact angle condition). While many existence and regularity results are known for solutions with zero contact angle, the only existence result with nonzero contact angle is due to Otto and only holds in some particular framework (Hele-Shaw cell). Following Bertsch, Giacomella and Karali, we take a different approach (regularization) to this free boundary problem to attempt to generalize Otto's result.