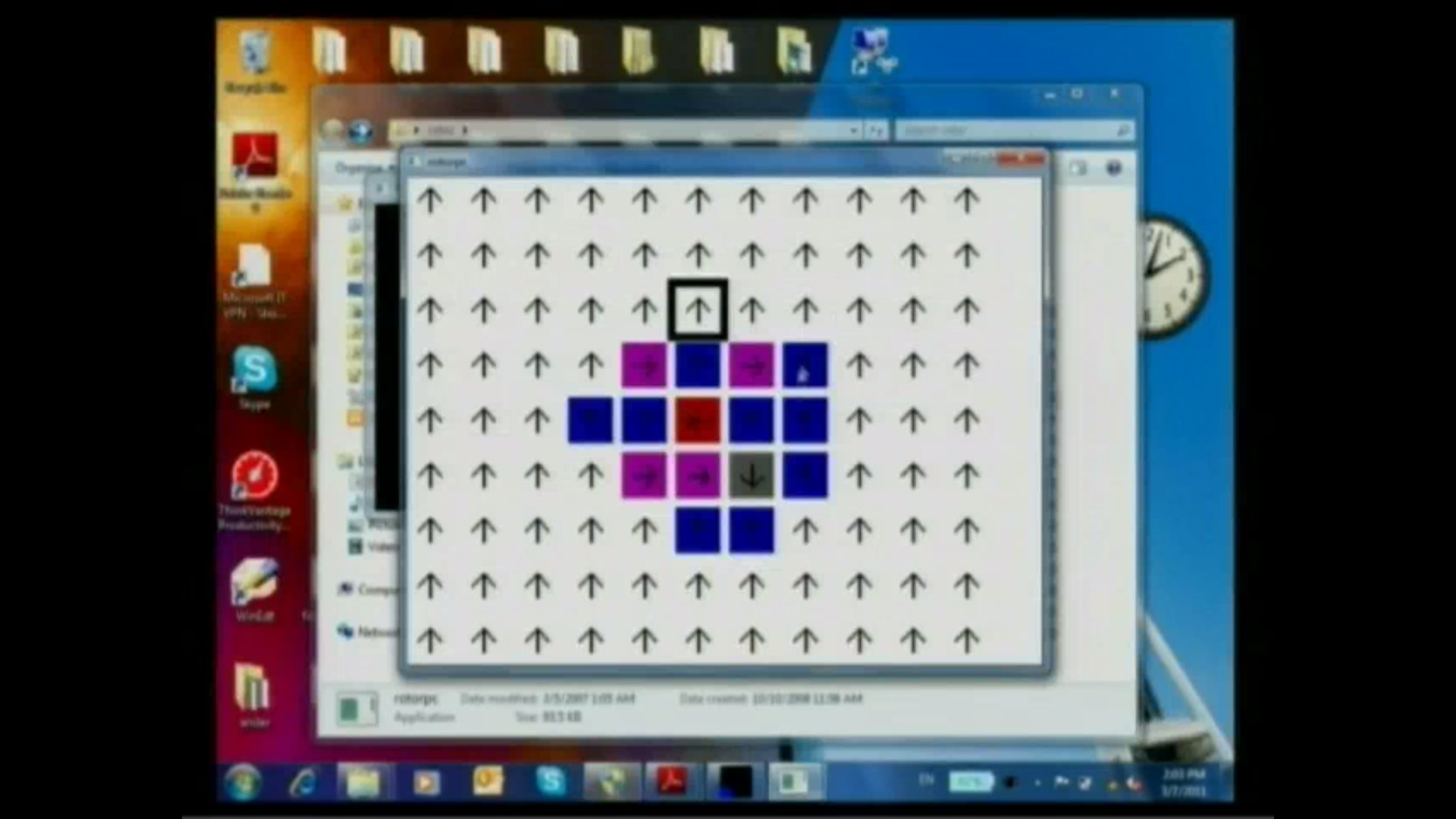
We compare several growth models on the two dimensional lattice. In some models, like internal DLA and rotor-router aggregation, the scaling limits are universal; in particular, starting from a point source yields a disk. Other sources yield a free-boundary problem for the Laplacian. In the abelian sandpile, particles are added at the origin and whenever a site has four particles or more, the top four particles topple, with one going to each neighbor. Despite similarities to other models, for the sandpile, the intriguing pattern that arises is not circular and depends on the particular lattice. It is an open problem to prove a scaling limit exists for the sandpile, though some bounds are known. I will describe some open problems, including a connection to conformal mapping which has not been established yet. Talk based on joint works with Lionel Levine.