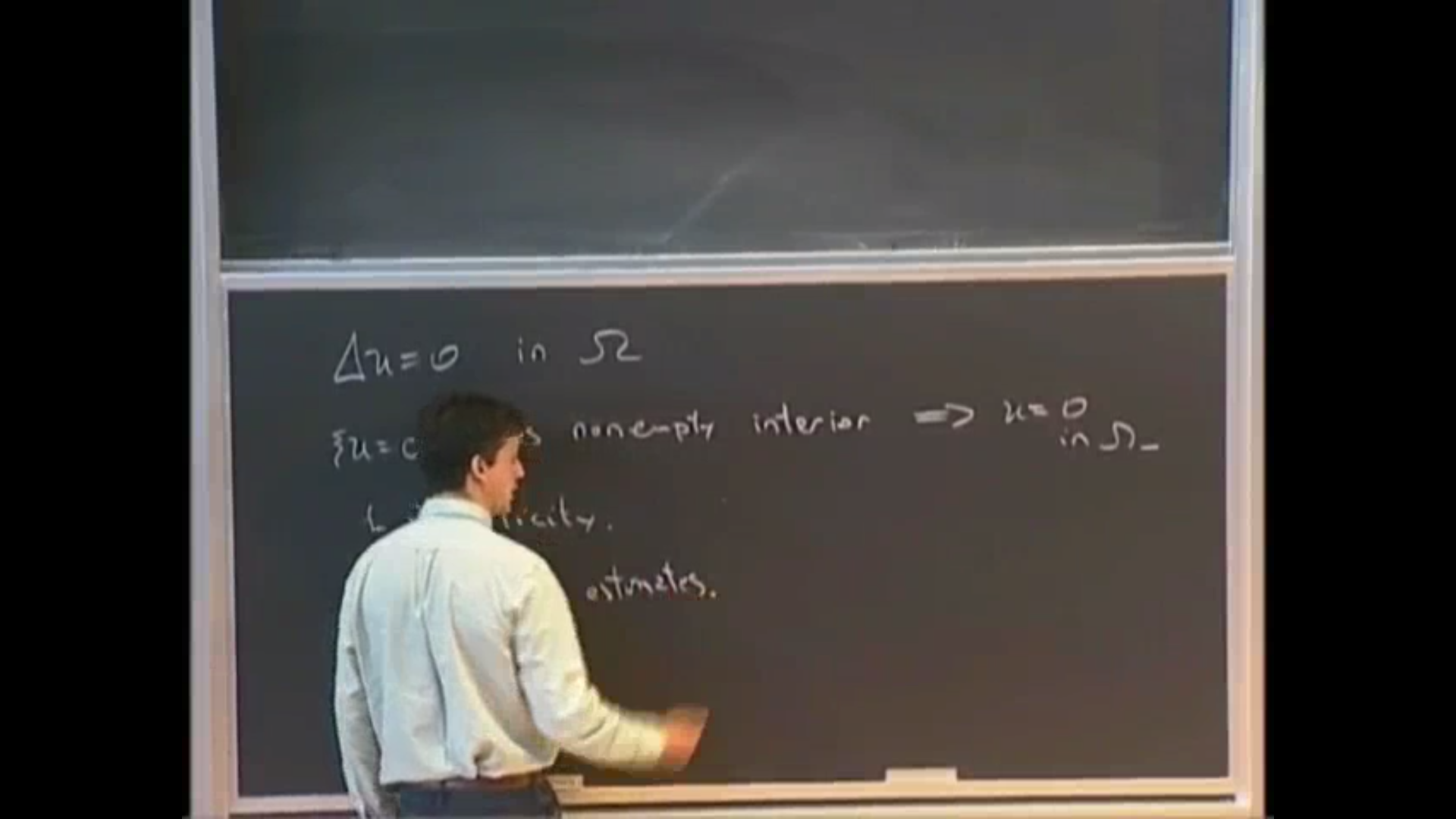We will discuss the following issue: if a solution to a nonlinear elliptic equation vanishes in a small ball, is it necessarily identically zero? The problem is fairly well-understood in the linear setting, but it is open for most interesting nonlinear elliptic equations. We will analyze the difficulties of the problem and prove a result in arguably the simplest case in which one cannot linearize the equation a priori. We will also relate it to a partial regularity result for fully nonlinear elliptic equations.