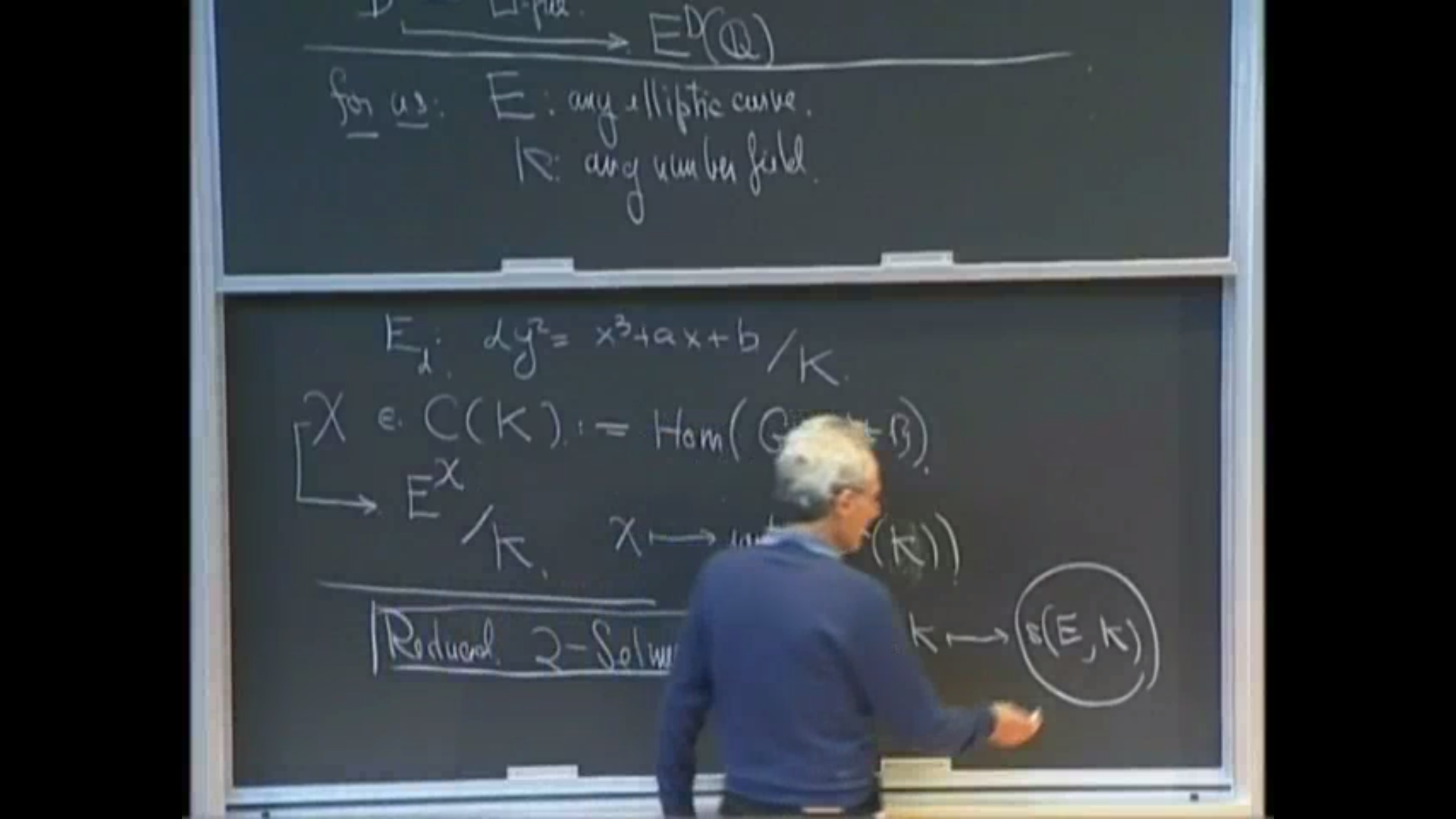Disparity in the statistics for quadratic twist families of elliptic curves
Presenter
April 13, 2011
Keywords:
- arithmetic geometry
- arithmetic statistics
- algebraic geometry over finite fields
- L-function
- twisted L-function
- character theory
- rational points
- asymptotic formulas for primes
- error estimates
- Selmer group
MSC:
- 11Gxx
- 11G40
- 11G30
- 11G20
- 11G45
- 11-xx
- 11Kxx
- 11Mxx
- 11K31
- 11K38
- 11K65
Abstract
(From attached notes)
The type of question we will examine has it roots in a famous result of Heath-Brown on the statistics of 2-Selmer ranks of a specific family of CM elliptic curves over Q related to the congruent number problem. This is the family E_D: Dy^2 = x^3-x for positive square-free integers D. The arithmetic of this family answers the question of whether or not D can be the common difference of an arithmetic progressions of squares of rational numbers.