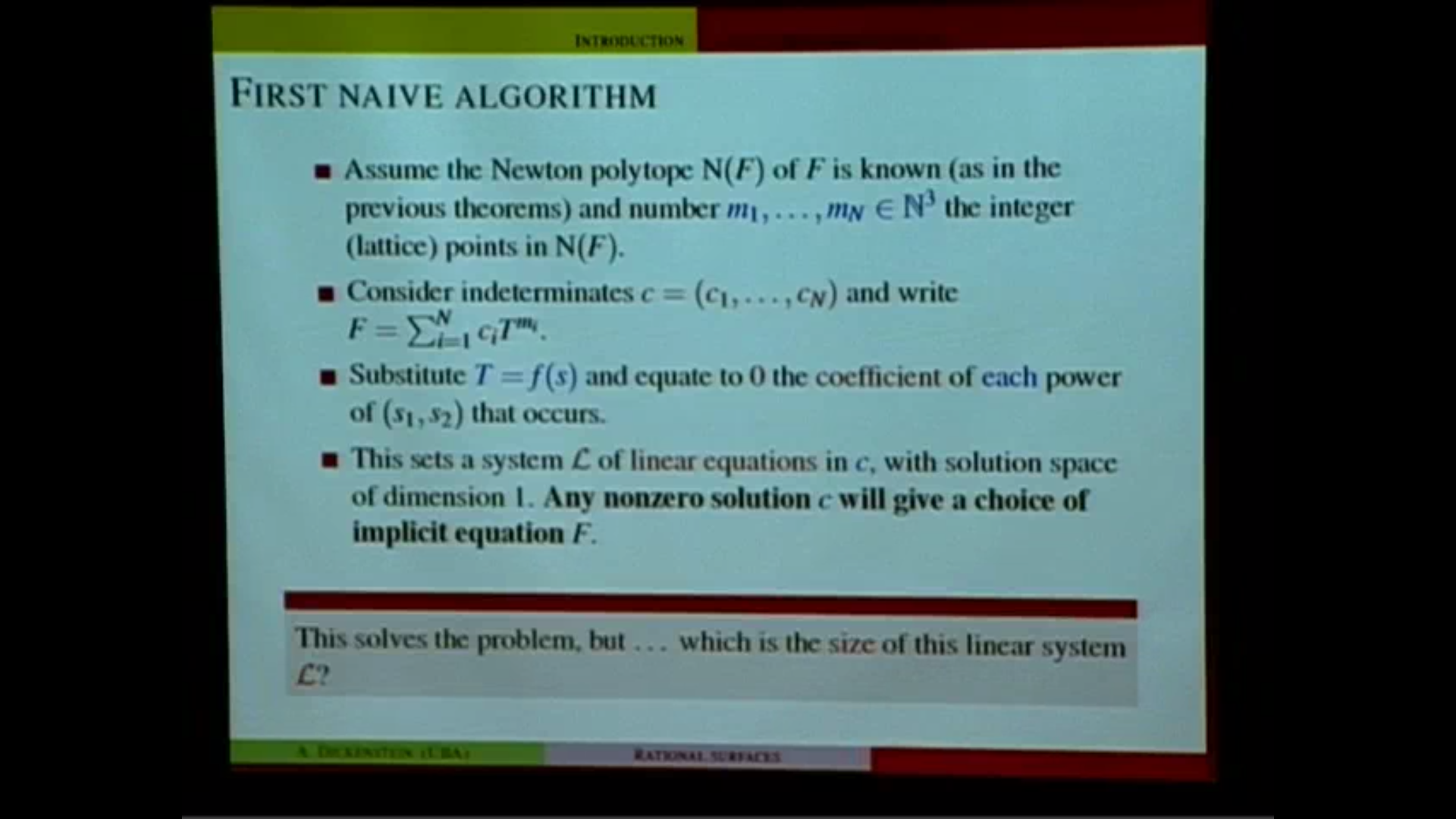
We will describe the use of linear syzygies for the implicitization of rational surfaces. These algebraic techniques, based on the theory of approximation complexes due to Jürgen Herzog, Aron Simis and Wolmer Vasconcelos, were introduced in this setting by Laurent Busé, Marc Chardin and Jean Pierre Jouanolou. Their work was inspired by the "practical" method of moving curves, proposed by Thomas Sederberg and Falai Chen. We will focus on exploiting the monomial structure of the polynomials defining the parametrization, following joint work with Nicolás Botbol and Marc Dohm, and we will concentrate in expressing the theoretical results into very concrete terms. Implementations in Macaulay2 were developed by Nicolás Botbol, Marc Dohm and Manuel Dubinsky.