Analytic Isomorphisms of Artin local K-algebras
Presenter
August 24, 2012
Keywords:
- algebraic combinatorics
- cluster algebra
- commutative algebra
- Artin algebras
- Macaulay's inverse system
- computational commutative algebra
- Hilbert scheme
- Poincare series
MSC:
- 13F60
- 13Fxx
- 13-xx
- 05-xx
- 16G10
- 16G50
- 16Gxx
- 16Kxx
Abstract
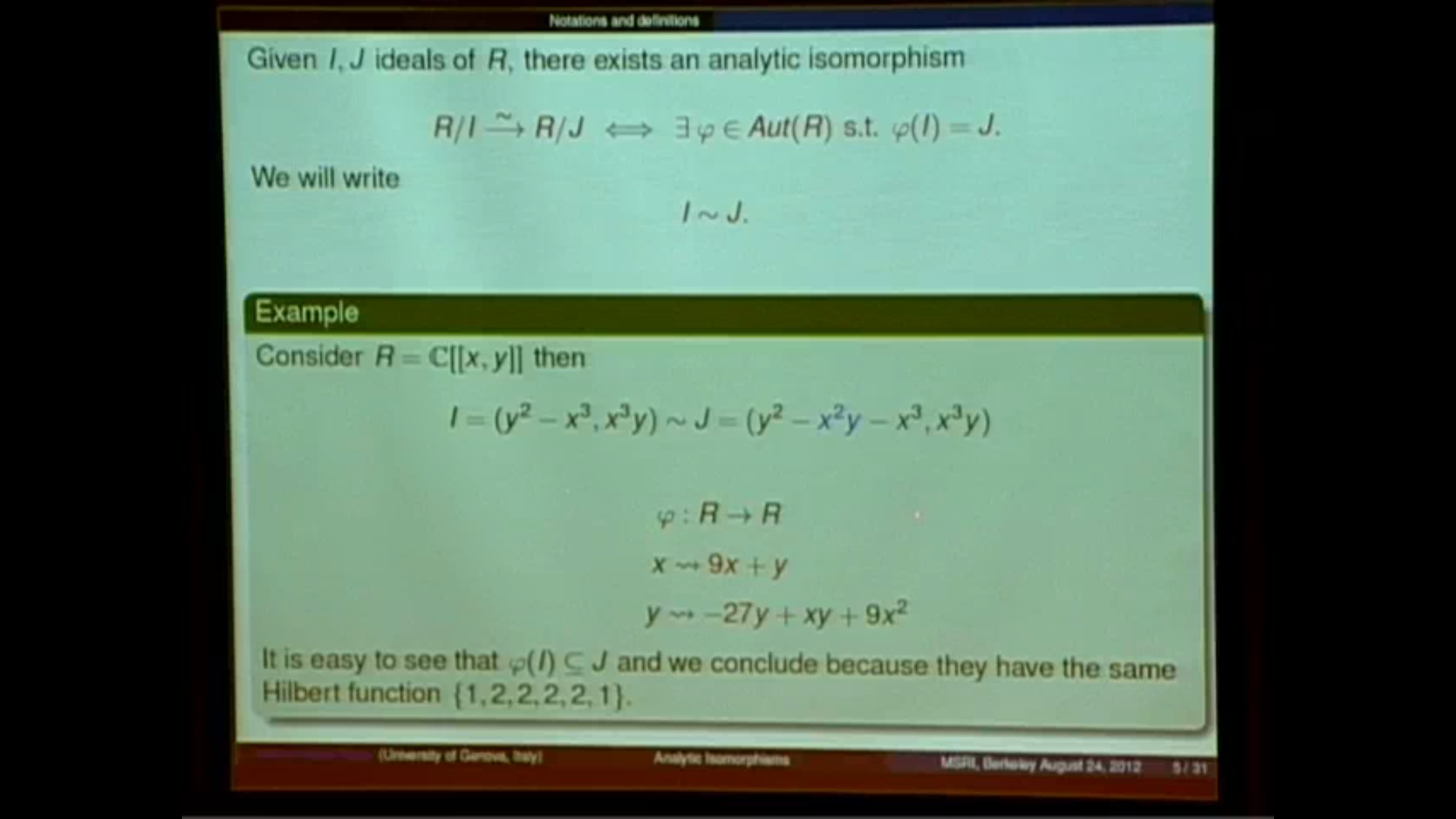
We will present recent results concerning isomorphism classes of Artin K-algebras through Macaulay's inverse system. By an effective computational framework we translate the problem in terms of the corresponding dual module in the polynomial ring. The aim is to prove that the study of certain classes of Artin local rings can be reduced to the study of standard graded K-algebras. In particular we prove that Artin local K-algebras having suitable socle degrees and maximal length (compressed algebras) are canonically graded, that is they are isomorphic to the corresponding associated graded ring as K-algebras. Examples show that the results cannot be generalized to any compressed K-algebra. We present some applications in problems concerning the punctual Hilbert scheme and the rationality of the Poincaré series.
- J.Elias, M.E. Rossi: "Isomorphism classes of short Gorenstein local rings via
Macaulay's inverse system", Trans. AMS, Vol 364, N.9 (2012), 4589-4604.
- J. Elias, M.E. Rossi: "Analytic Isomorphisms of compressed local algebras", preprint
(2012)