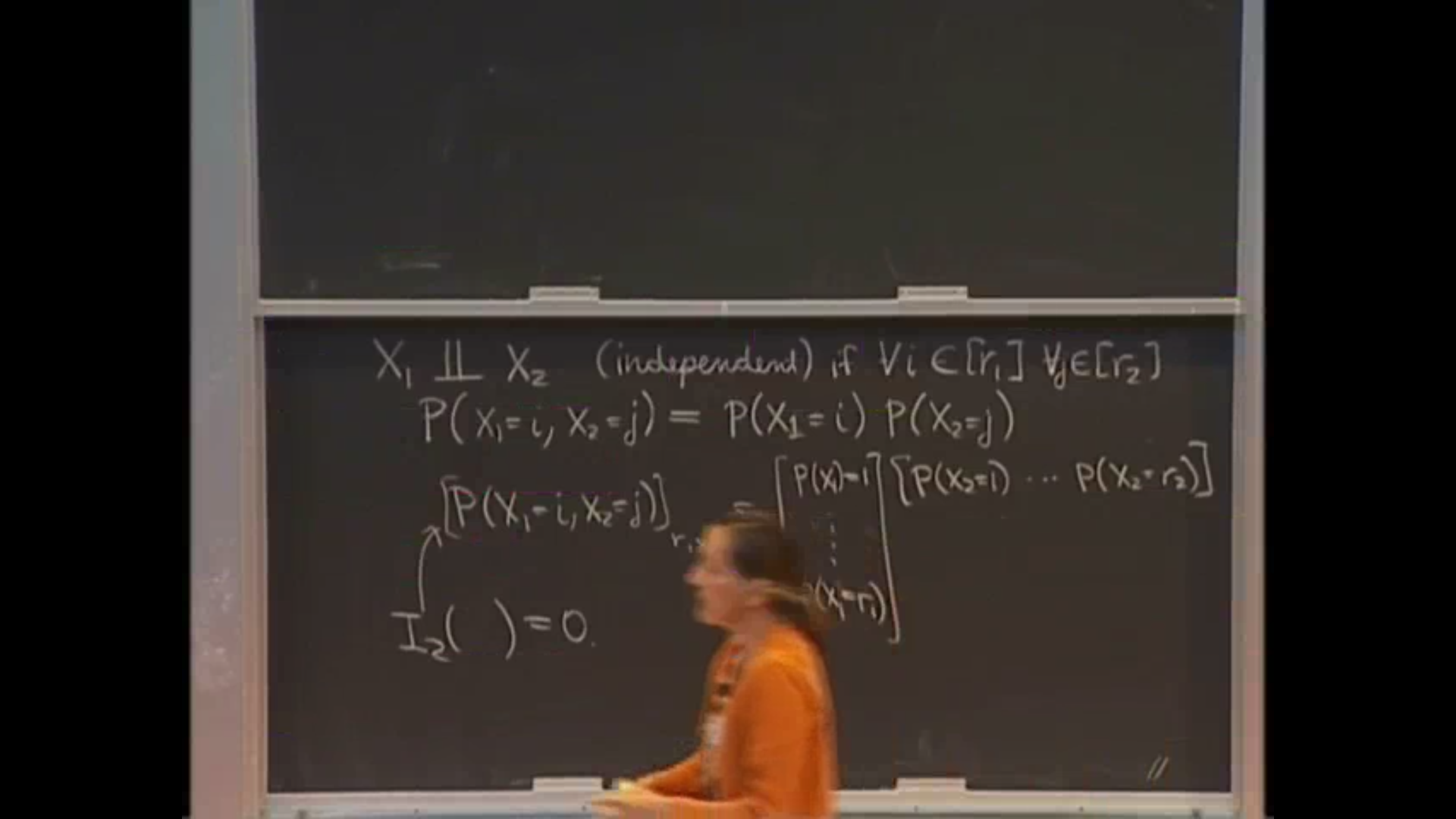Minimal components over certain binomial ideals
Presenter
August 23, 2012
Keywords:
- algebraic combinatorics
- commutative algebra
- cluster algebra
- binomial ideals
- minimal primes
- algebraic statistics
- probability theory
- hyper-matrices
- polynomial rings
MSC:
- 13F60
- 13Fxx
- 13-xx
- 05-xx
- 16S34
- 05A10
- 05A15
Abstract
This is joint work with Amelia Taylor, Julia Porcino, and Alessio Sammartano.
The main motivation of this work was to understand Alex Fink's paper on minimal components arising in algebraic statistics.
With Taylor we generalized Fink's work to any finite number of discrete random variables, and we expressed the minimal components of the ensuing determinantal ideals in terms of some combinatorial structures. With Porcino we instead looked at the corresponding permanental ideals, and again expressed the minimal components with different combinatorial structures. The hypermatrices on which these determinantal and permanental ideals are defined are generic.
Sammartano instead took s-catalecticant hypermatrices, with s a parameter varying over integers from 1 to the the dimension of the hypermatrix; 1-catalecticant hypermatrices are generic, and for s > 1 the s-catalectican hypermatrices are progressively less generic. Sammartano obtained the combinatorial structure of the minimal components in these cases as well.