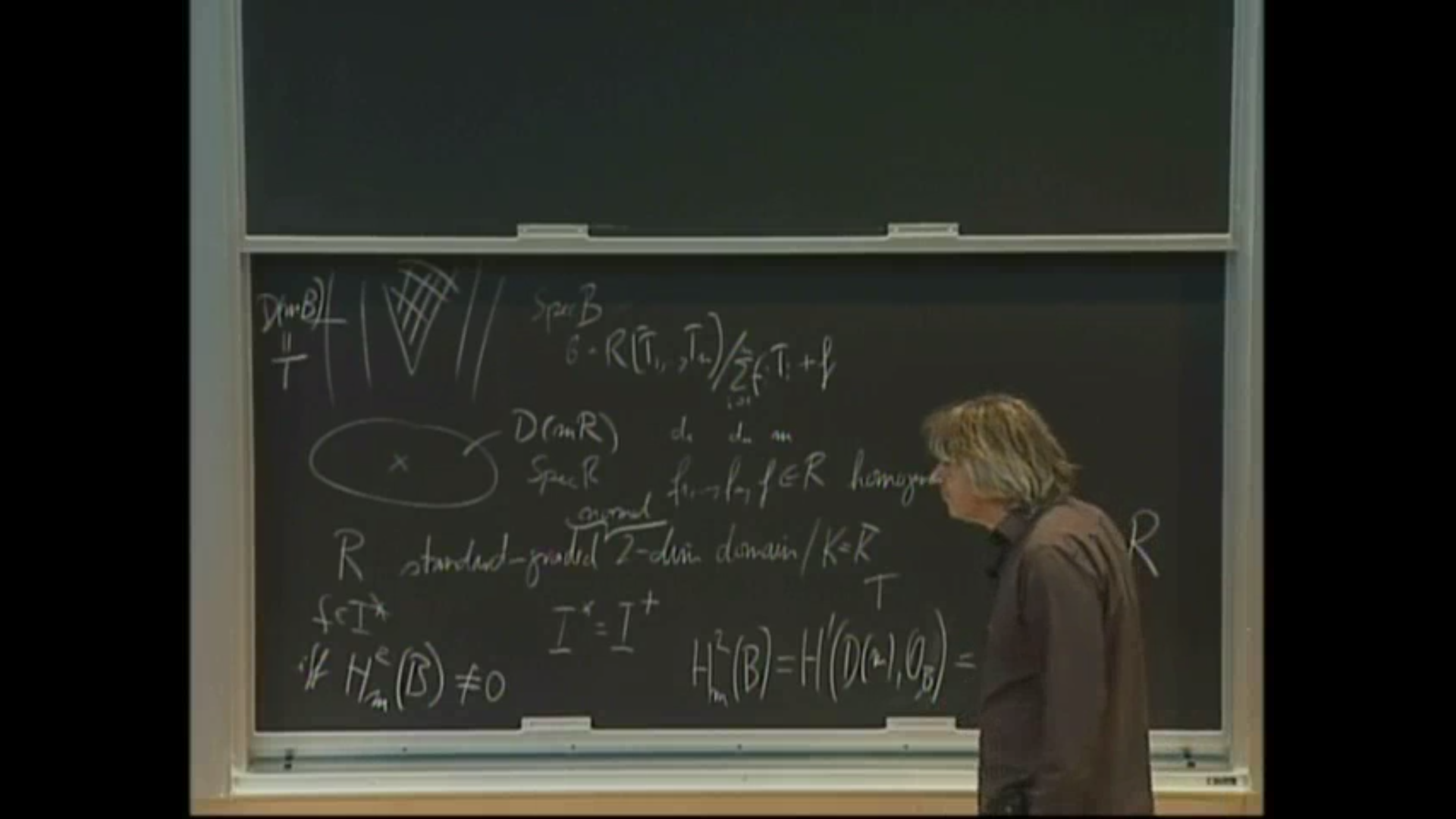In this course, we will discuss how ideal closure operations can be understood by studying the interplay between forcing algebras, vector bundles, and their torsors. A torsor is a nice geometric model of a first cohomology class. This interplay works best when the closure operation depends only on the cohomology class, which is true for tight closure, plus closure, and Frobenius closure under mild conditions. Special emphasis will be given to the case of a graded normal ring of dimension two, where we can work over the corresponding smooth projective curve and may use established methods and results (like the existence of moduli spaces for semistable bundles) to obtain new theorems and interesting examples for these closure operations.