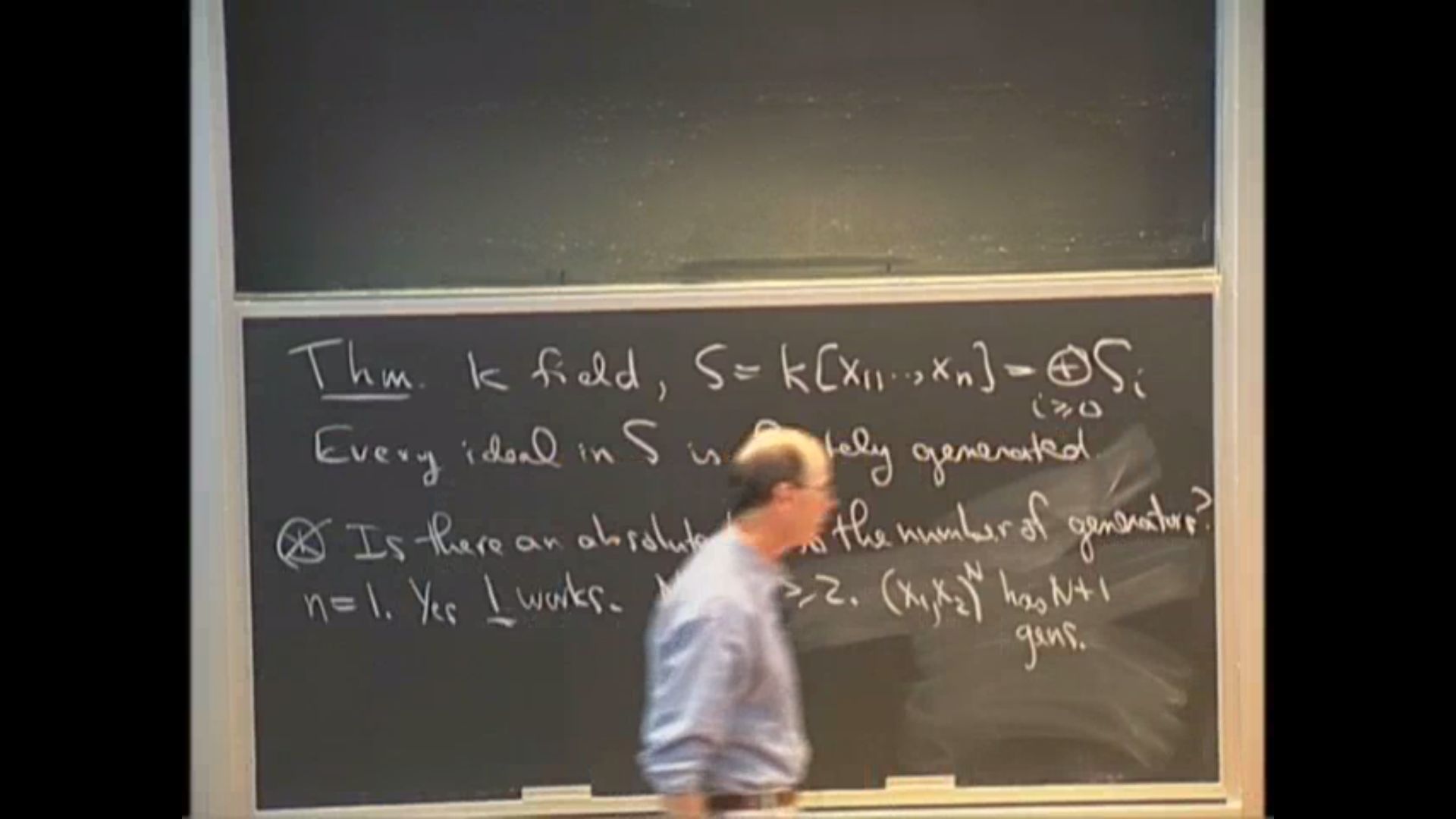It can be argued that two of the most important theorems in commutative algebra were proved by Hilbert: the Hilbert Basis Theorem (Frank Schreyer will be giving a proof of this theorem) and the Hilbert Syzygy theorem. We will review these theorems as some of the first avatars of the theme of uniformity in commutative algebra, and use them to introduce some basic concepts such as projective dimension, regularity, Cohen-Macaulayness, and multiplicity. The first lecture will discuss resolutions and some of conjectured uniformity results on regularity and projective dimension. Irena Peeva will be speaking in much more detail concerning infinite resolutions.