Combinatorics of KP solitons from the real Grassmannian
Presenter
October 31, 2012
Keywords:
- commutative algebra
- cluster algebra
- algebraic combinatorics
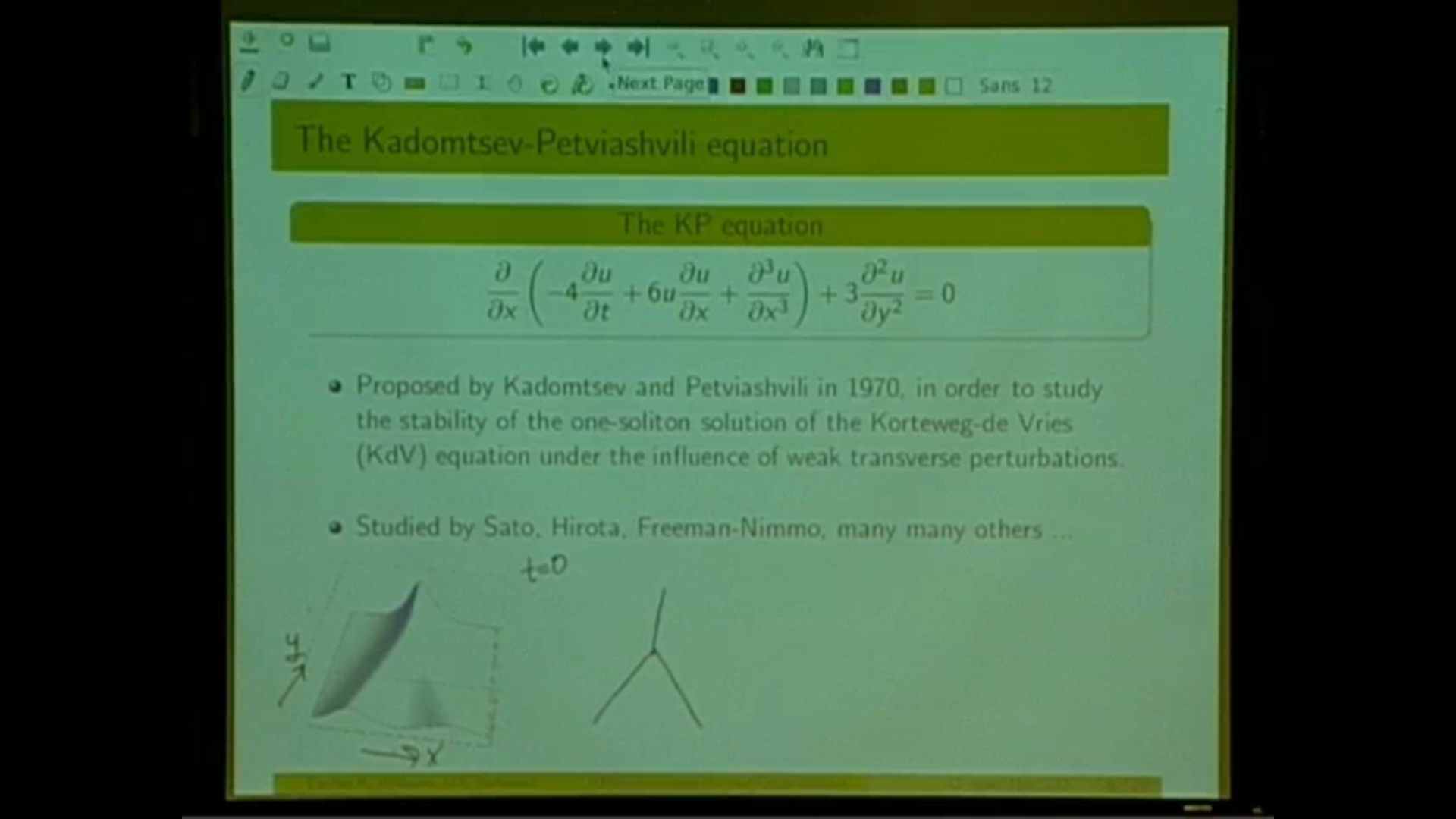
- KP equations
- regularity
- positivity
- soliton solutions
- tropical geometry
- Grassmannians
- surface waves
MSC:
- 13F60
- 13F55
- 13Fxx
- 13-xx
- 05-xx
- 06-xx
- 37K10
- 37K40
- 37K30
- 37K45
- 37Kxx
- 37-xx
- 37-xx
Abstract
Given a point A in the real Grassmannian, it is well-known that one can construct a soliton solution u_A(x,y,t) to the KP equation. The contour plot of such a solution provides a tropical approximation to the solution when the variables x, y and t are considered on a large scale and the time t is fixed. I will describe joint work with Yuji Kodama on the combinatorics of such contour plots. Using the positroid stratification and the Deodhar decomposition of the Grassmannian (and in particular the combinatorics of Go-diagrams), we completely describe the asymptotics of these contour plots when |y| or |t| go to infinity. Other highlights include: a surprising connection with total positivity and cluster algebras, and the characterization of regular soliton solutions -- that is, a soliton solution u_A(x,y,t) is regular for all times t if and only if A comes from the totally non-negative part of the Grassmannian. No background on the KP equation or the Grassmannian will be required.