Relations Between Minors (joint with Winfried Bruns and Aldo Conca)
Presenter
December 6, 2012
Keywords:
- commutative algebra
- algebraic combinatorics
- Stanley-Reisner rings
- representation theory
- determinantal variety
- syzygies
- Young diagram
- Schur modules
- Schur-Weyl duality
- minimal relations
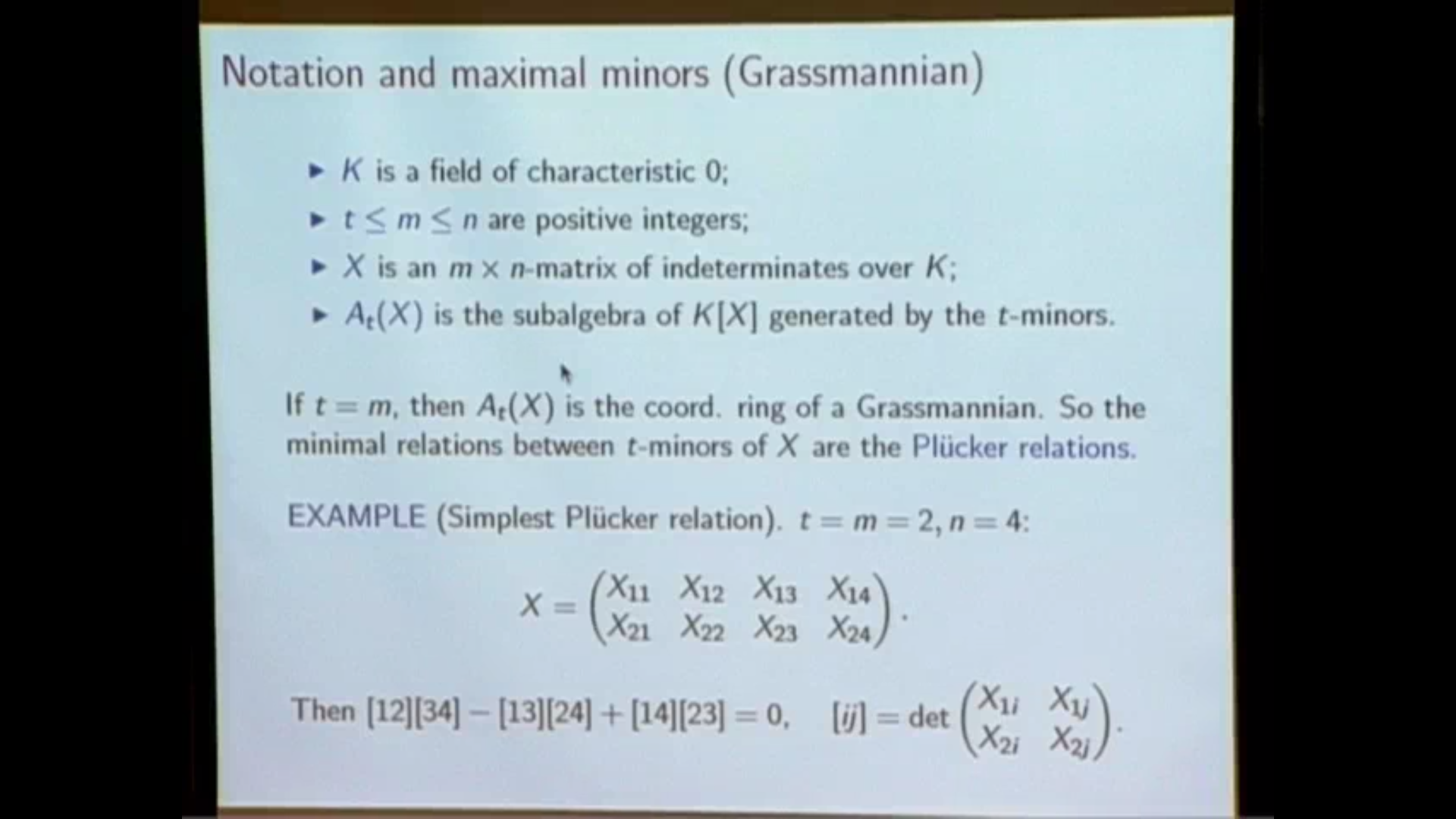
- Grassmannians
- Plucker relations
MSC:
- 05-XX
- 05EXX
- 05E15
- 05E40
- 14M15
- 14M12
- 14M10
- 14M05
- 14M20
- 14M25
- 14Mxx
- 14-xx
Abstract
It is a classical theorem that the minimal relations between the maximal minors of a generic matrix are the Plücker relations. In particular they are quadratic. Surprisingly, the relations of t-minors (t fixed) are, in general, not understood at all. The goal of the talk is to explain the representation theoretic aspect of the problem that leads to a description of some natural polynomials vanishing on t-minors. We propose the conjecture that all the relations can be generated by them. In particular, it would follow that quadrics and cubics are enough to generate the ideal of relations between minors.
Some evidence of this conjecture will be reported.