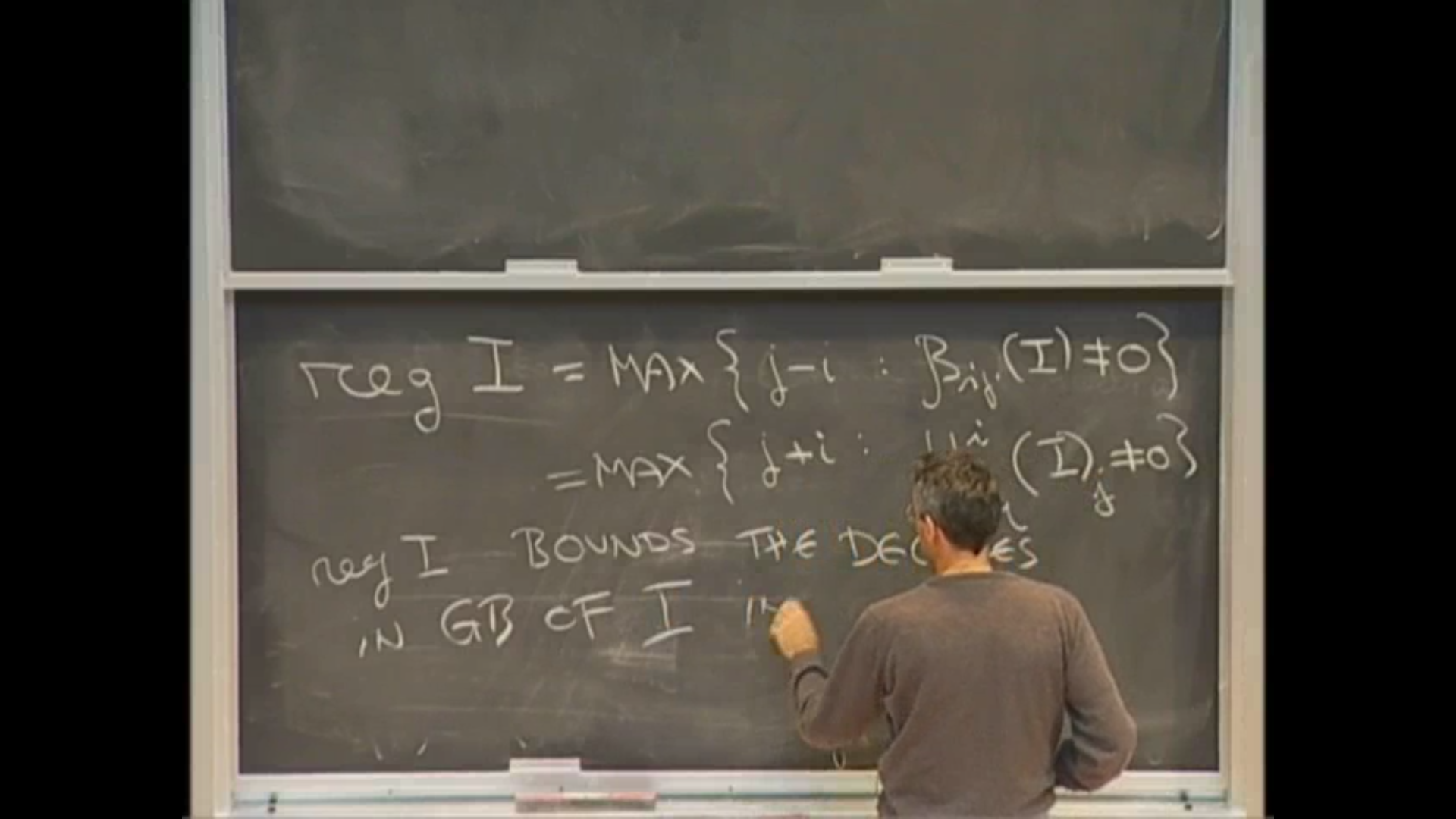The Castelnuovo-Mumford regularity of the powers I^h of a homogeneous ideal I in the polynomial ring is asymptotically a linear function of h. Clearly reg(I^h) is at least hm where m is the smallest degree of a generator of I. We say that I has linear powers if reg(I^h)=hm, that is, if all the powers of I have a linear resolution. We show that the ideal of maximal minors of a sufficiently general matrix with linear entries has linear powers. In particular we prove that every rational normal scroll has linear powers. This is a joint work with Winfried Bruns and Matteo Varbano, see arXiv:1203.1776.