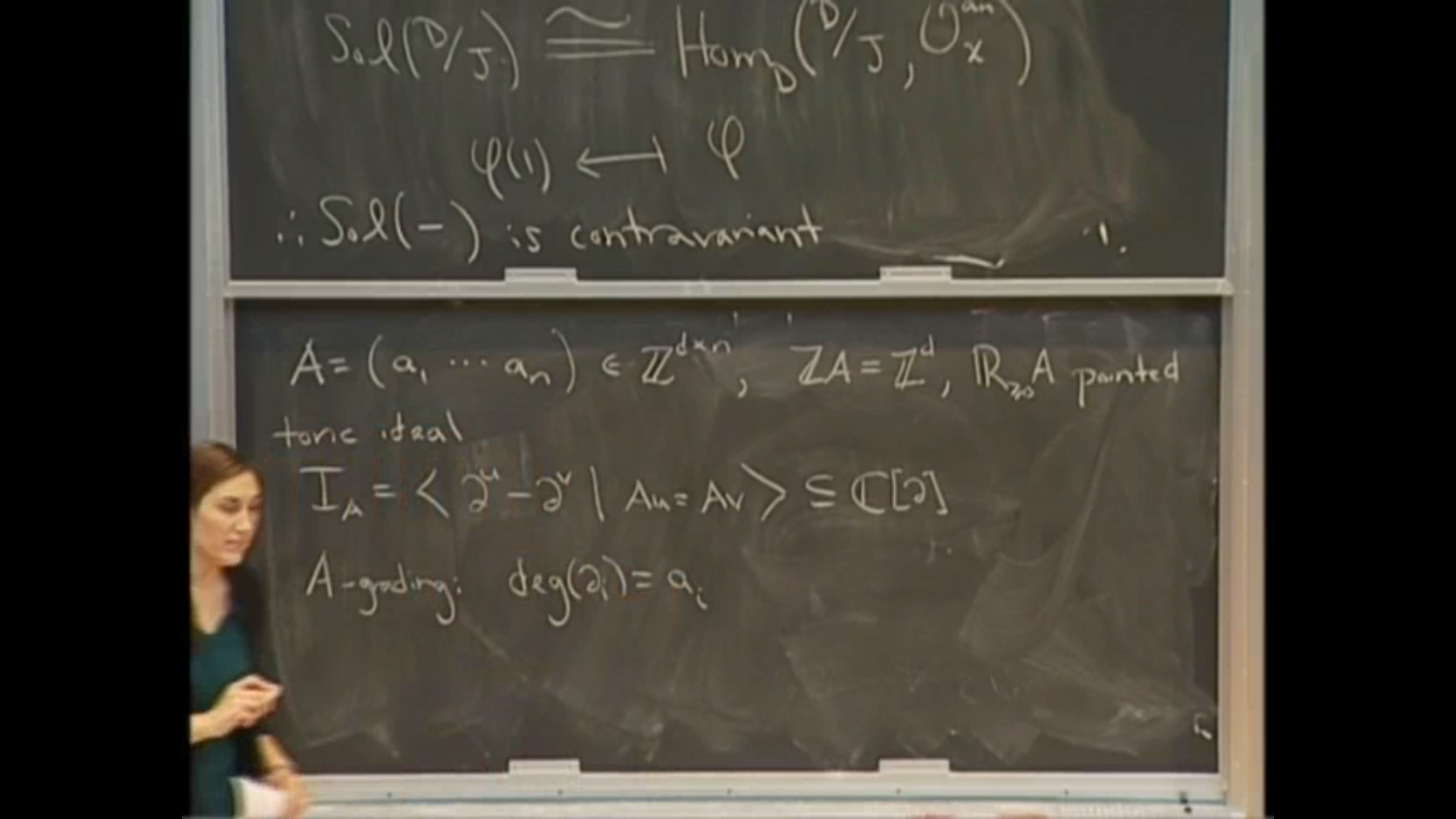Euler--Koszul homology for hypergeometric systems
Presenter
December 5, 2012
Keywords:
- commutative algebra
- algebraic combinatorics
- Stanley-Reisner rings
- A-hypergeometric system
- D-modules
- toric varieties
- Euler operators
- rank jumps
- GKZ-hypergeometric system
MSC:
- 05-XX
- 05EXX
- 05E15
- 05E40
- 16S37
- 32C38
- 14M25
Abstract
A-hypergeometric systems are the D-module counterparts of toric ideals, and their behavior is linked closely to the combinatorics of toric varieties. Euler-Koszul homology is a D-module Koszul functor introduced by Matusevich, Miller and Walther, that has shed great light on these systems. We will discuss its implications for the parametric behavior of their solution spaces.