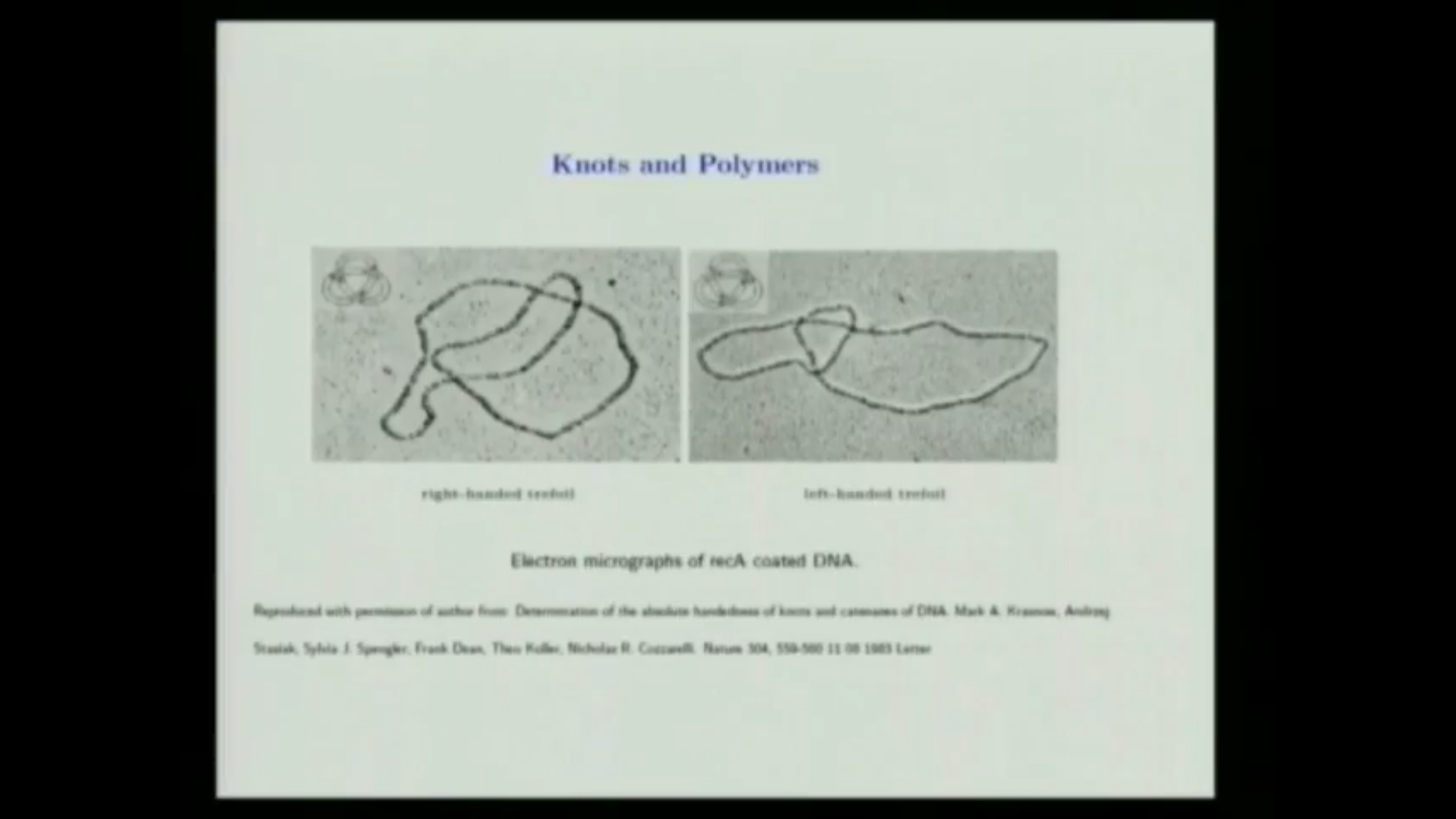
With the goal of understanding polymer entanglements, for over 20 years there has been interest in questions about knotting and linking of self-avoiding polygons on the simple cubic lattice. Notably, in 1988 Sumners and Whittington proved that all but exponentially few sufficiently long self-avoiding polygons are knotted. This proved the long standing Frisch-Wasserman-Delbruck conjecture that sufficiently long ring polymers will be knotted with high probability. Since then there has been progress both theoretically and numerically using lattice models to investigate polymer entanglements. Much of this progress has been motivated by questions arising from the study of DNA topology. At the same time many open questions remain. I will review progress made and highlight open problems especially in the context of extensions of the Sumners and Whittington theoretical approach to questions about knotting and linking in systems of self-avoiding polygons and walks.