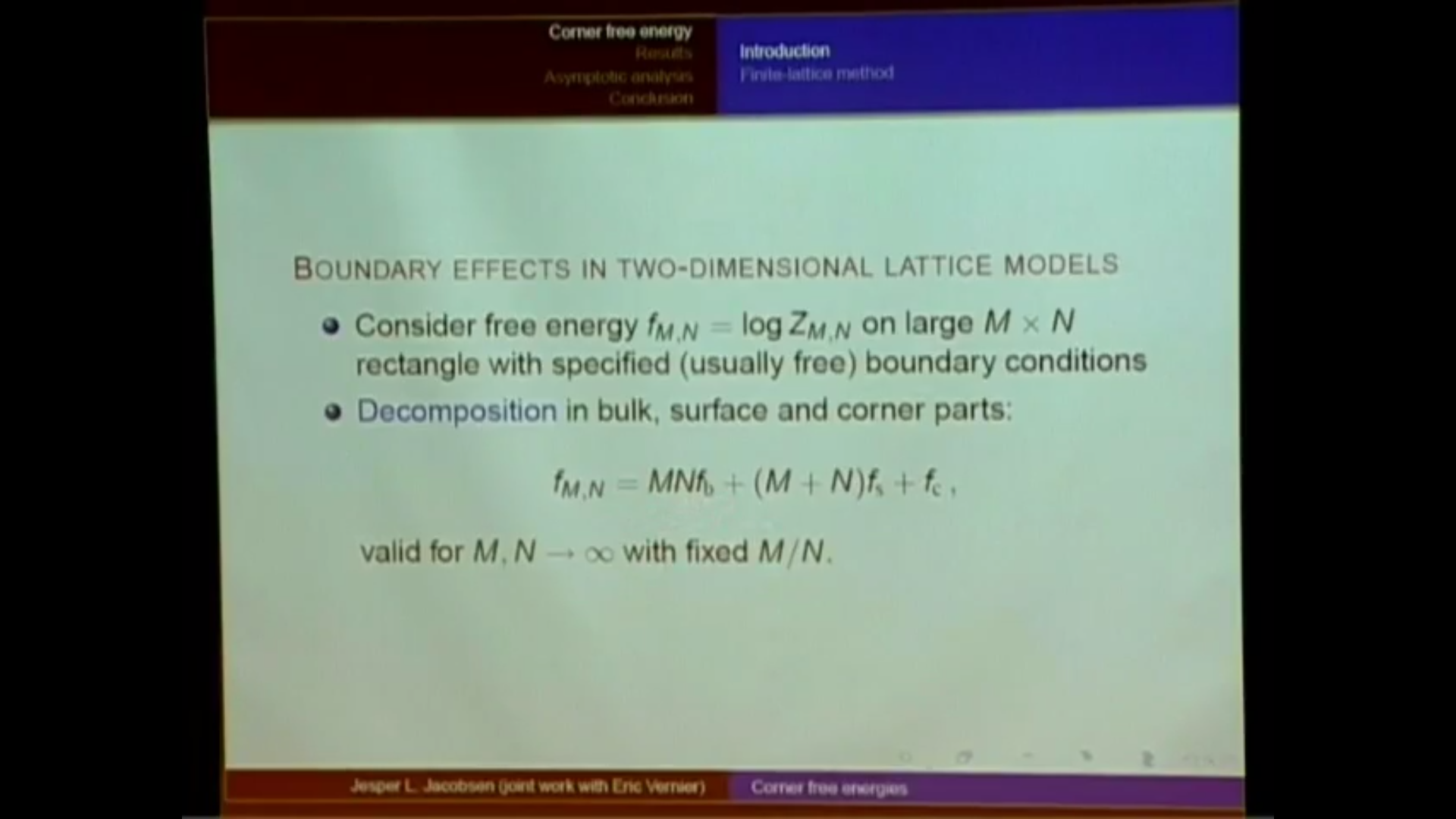
We obtain long series expansions for the bulk, surface and corner free energies for several two-dimensional statistical models. The models encompass all integrable curves of the Q-state Potts model on the square and triangular lattices, including the antiferromagnetic transition curves and the Ising model (Q=2) at temperature T, as well as a fully-packed O(n) type loop model on the square lattice. All expansions turn out to have the form of infinite products with a certain periodicity property, enabling us to conjecture the form of the expansions to all orders. We analyze in detail the limits in which the models become critical. In this limit the divergence of the corner free energy defines a universal term which can be compared with the conformal field theory (CFT) predictions of Cardy and Peschel. This allows us to deduce the asymptotic expressions for the correlation length in several cases.