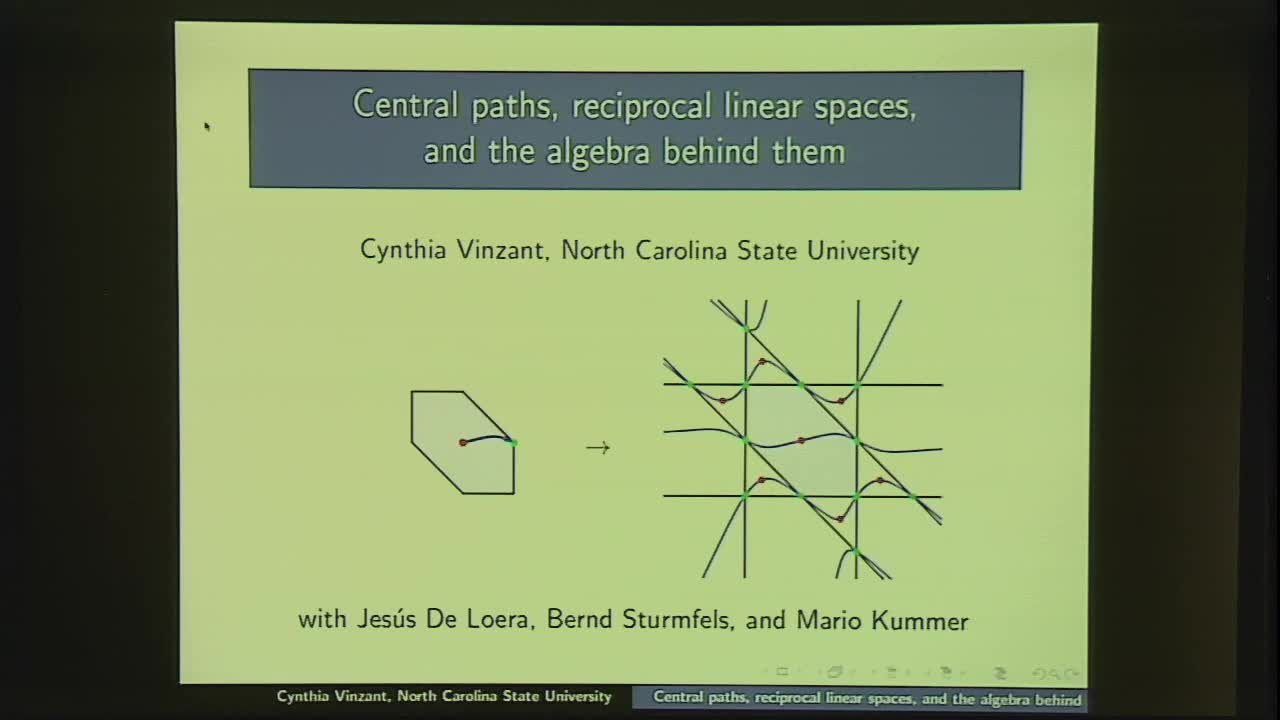
Analytic Centers, Reciprocal Linear Spaces, and Planes that Intersect Them
Presenter
February 24, 2015
Keywords:
- Linear algebra
Abstract
In interior point methods for linear programming, the central path starts at the analytic center of a polytope. The collection of analytic centers corresponding to a given constraint matrix form an algebraic variety called a reciprocal linear space. We investigate this variety through its Chow form, namely the hypersurface in the Grassmannian of planes that intersect it. This hypersurface has a beautiful representation as the determinant of a symmetric matrix, which reveals real algebraic properties of this collection of analytic centers.