Under rapid periodic pacing, cardiac cells typically undergo a period-doubling bifurcation in which action potentials of short and long duration alternate with one another. If these action potentials propagate in a fiber, the short-long alternation may suffer abrupt reversals of phase at various points along the fiber, a phenomenon called (spatially) discordant alternans. Either stationary or moving patterns are possible. 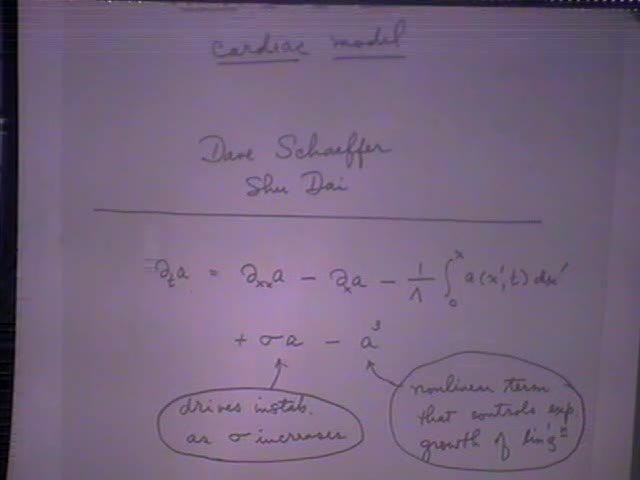Echebarria and Karma proposed an approximate equation to describe the spatiotemporal dynamics of small-amplitude alternans in a class of simple cardiac models, and they showed that an instability in this equation predicts the spontaneous formation of discordant alternans. We show that for certain parameter values a degenerate steady-state/Hopf bifurcation occurs at a multiple eigenvalue. Generically, such a bifurcation leads one to expect chaotic solutions nearby, and we perform simulations that find such behavior. Chaotic solutions in a one-dimensional cardiac model are rather surprising--typically chaos in the cardiac system has occurred from the breakup of spiral waves in two dimensions.