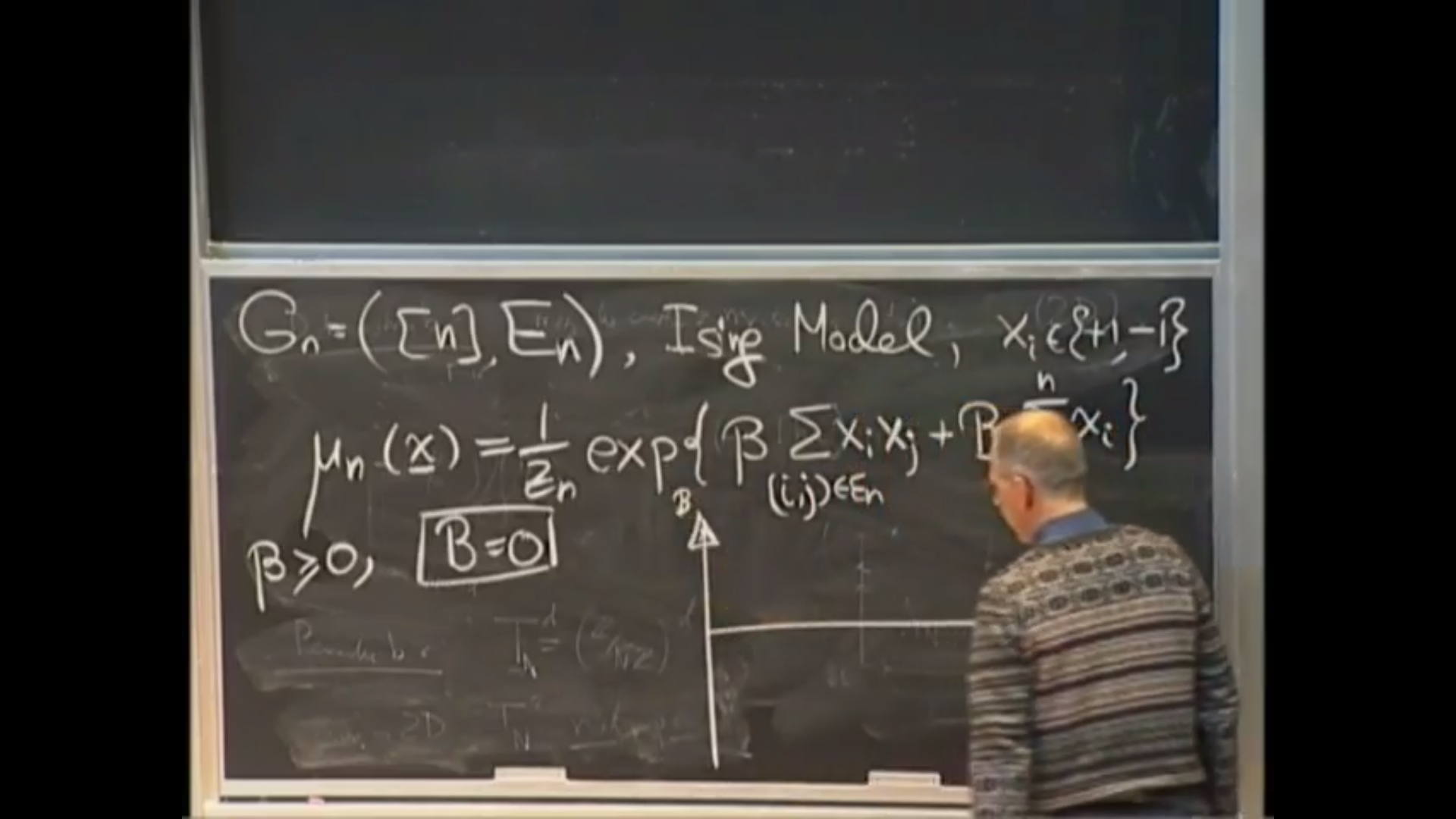Ferromagnetic Ising measures on large locally tree-like graphs
Presenter
February 20, 2012
Keywords:
- mathematical statistical mechanics
- percolation
- probability theory
- phase transitions
- scaling laws
- SLE
- Ising model
- ferromagnetism
- graph theory
- expander graph
MSC:
- 82C20
- 82C05
- 82C44
- 82C43
- 82C26
- 82C27
- 82Cxx
- 82-xx
- 82D45
Abstract
Consider the ferromagnetic Ising measure on sparse finite graphs converging locally to limiting tree T. In case T is d-regular, it was recently shown by Montanari, Mossel and Sly that these Ising measures converge locally to symmetric mixture of plus and minus (boundary conditions) Ising measures on T, and for expander graphs, conditioned on positive magnetization these measures converge to plus-boundary condition Ising measure on T.
In this talk, based on a joint work with Anirban Basak, I will review this result and explain what happens for more general, random limiting tree T.