Conformal blocks in 2d CFT, the Calogero-Sutherland model and the AGT conjecture
Presenter
March 30, 2012
Keywords:
- mathematical physics
- mathematical statistical mechanics
- conformal field theory
- conformal invariance
- probability theory
- random point process
- SLE
- fractional quantum Hall effect
- nonabelian statistics
- integrable systems
- Virasoro algebra
MSC:
- 60K35
- 60J45
- 60J65
- 60J67
- 60Jxx
- 60-xx
- 60G57
- 60G60
- 82-xx
- 82B44
- 82B43
- 81R10
- 81R12
- 81Rxx
Abstract
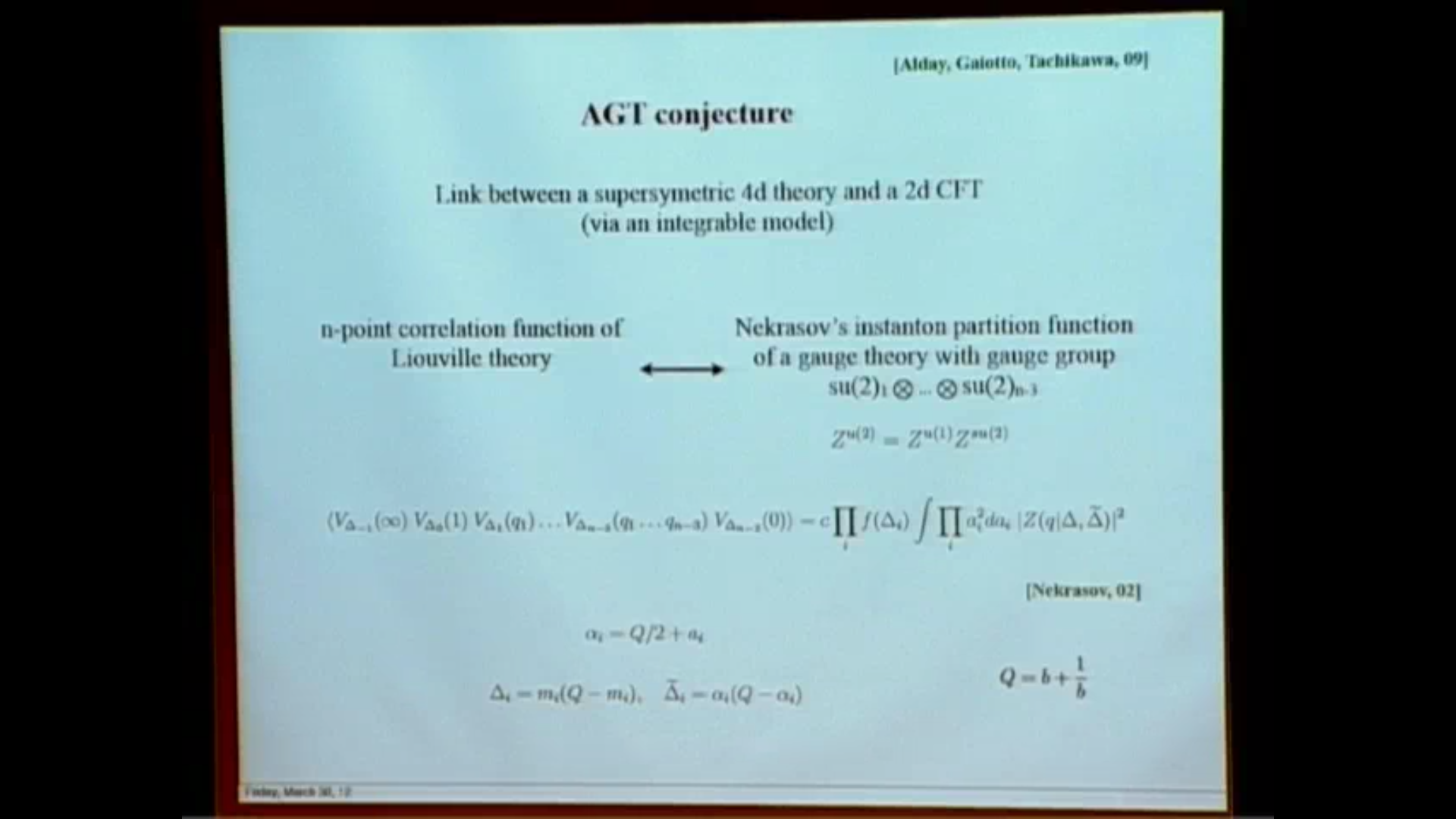
There is renewed interest in the properties of the conformal blocks of 2d conformal field theories, due to their relation with the physics of the Fractional Quantum Hall Effect and with the Alday, Gaiotto and Tachikawa conjecture. We have discovered that there is a natural action of the Calogero-Sutherland Hamiltonian on the conformal blocks containing second-order degenerate operators, and that this action can be related to the structure discovered by Alba, Fateev, Litvinov and Tarnopolsky in Liouville theory in the context of the AGT conjecture. Higher order integrals of motion can be associated to higher order degenerate fields. I will explain this construction for the conformal field theories with Virasoro and W-extended symmetry.