Conformal invariance of spin correlations in the planar Ising model
Presenter
March 29, 2012
Keywords:
- mathematical physics
- mathematical statistical mechanics
- conformal field theory
- conformal invariance
- probability theory
- random point process
- SLE
- Ising model
- correlation function
MSC:
- 60K35
- 60J45
- 60J65
- 60J67
- 60Jxx
- 60-xx
- 60G57
- 60G60
- 82-xx
- 82B44
- 82B43
- 82B20
Abstract
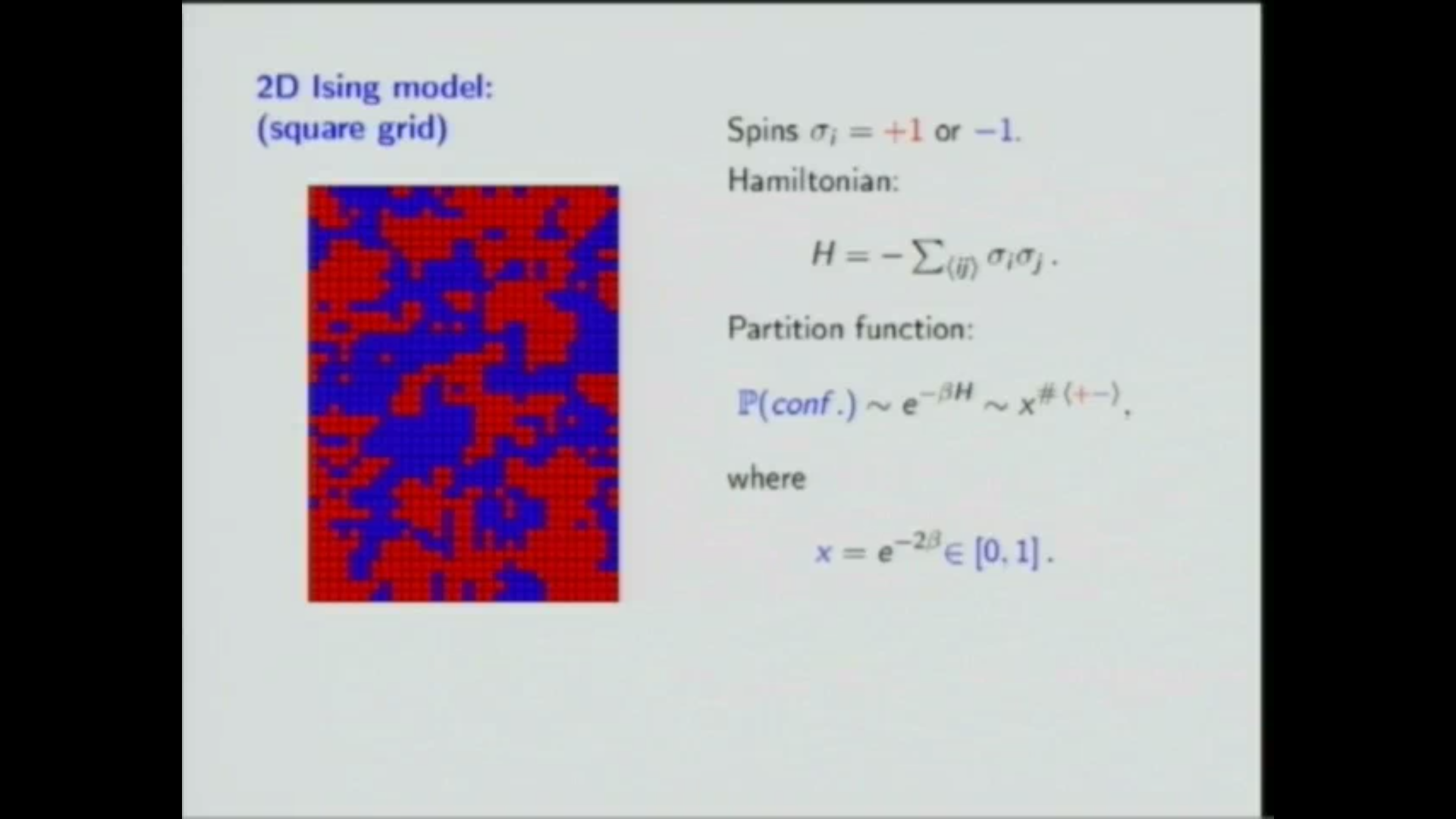
We rigorously prove existence and conformal covariance of scaling limits of spin correlations in the critical Ising model (defined on square grid approximations of a simply connected planar domain). This solves a number of conjectures coming from physical and mathematical literatures. The proof is based on convergence results for discrete holomorphic spinor observables which allow us to compute the logarithmic derivatives of those correlations with respect to positions of points, and relate the correlations for various boundary conditions to each other.
Based on a joint work with Clement Hongler and Konstantin Izyurov (arXiv:1202.2838 and arXiv:1105.5709)