Taming the Integrable Zoo
Presenter
March 29, 2012
Keywords:
- mathematical physics
- mathematical statistical mechanics
- conformal field theory
- conformal invariance
- random point process
- probability theory
- SLE
- discrete holomorphicity
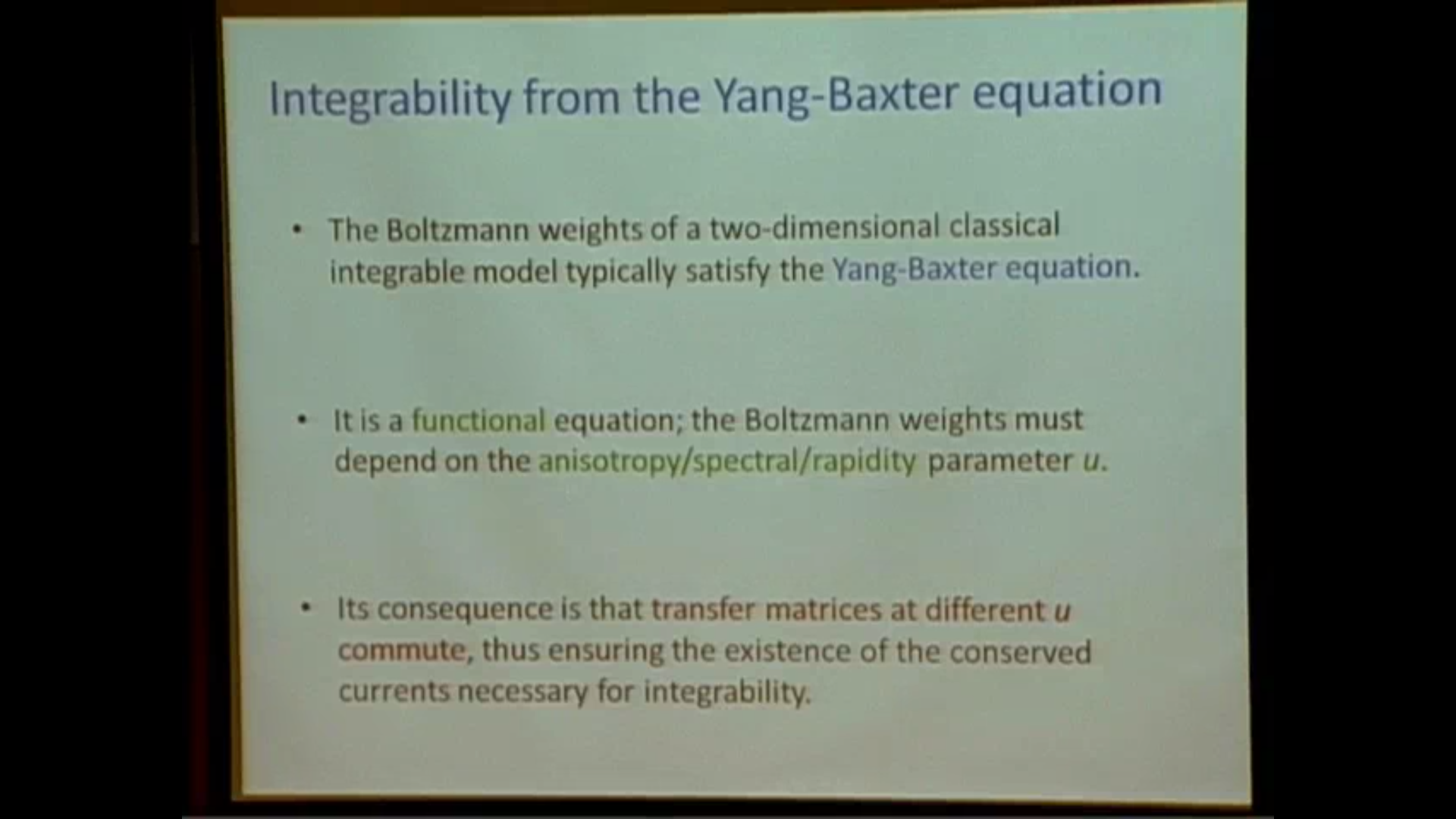
- Yang-Baxter equation
- integrable systems
- Jones polynomial
MSC:
- 60K35
- 60J45
- 60J65
- 60J67
- 60Jxx
- 60-xx
- 60G57
- 60G60
- 82-xx
- 82B43
- 82B44
Abstract
Two-dimensional critical integrable models have long been known to be intimately connected to three-dimensional topology. For example, knot and link invariants such as the Jones polynomial can be obtained as a certain limit of Boltzmann weights satisfying the (non-linear) Yang-Baxter equation. Recently, Cardy and collaborators have shown that such Boltzmann weights often can be obtained from a set of linear relations ensuring that certain operators are discretely holomorphic. I explain how discrete holomorphicity naturally arises by defining these operators in terms of three-dimensional link invariants. This allows the results of Smirnov and Cardy et al to be extended both to lattice height models, and to new hierarchies of integrable models (e.g. those associated with the Birman-Wenzl-Murakami algebras). It also provides a direct connection between integrability and conformal field theory.