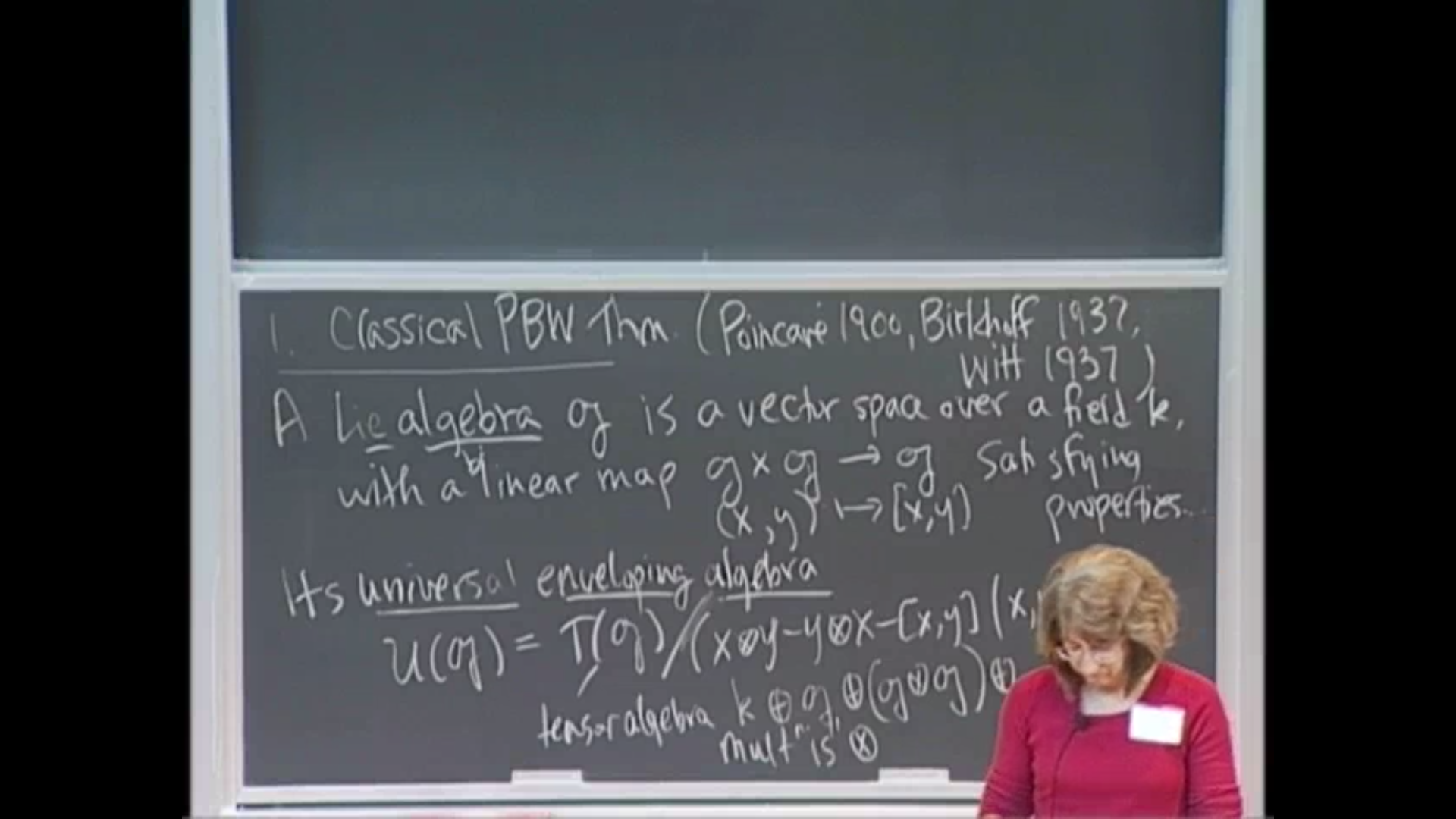The classical Poincare-Birkhoff-Witt (PBW) theorem sheds light on the structure of a Lie algebra: it embeds into an associative algebra, namely its universal enveloping algebra, that behaves in some ways like a polynomial ring. More precisely, the universal enveloping algebra is filtered with its associated graded algebra a polynomial ring. Many other algebras share this advantageous property. In particular, they have PBW bases, which greatly facilitate their study. Examples include symplectic reflection algebras and graded Hecke algebras. In this talk, we will discuss PBW theorems in the context of some of these algebras of current interest. We will note some recent developments, particularly in positive characteristic.