Recent progress on geometric analysis and Riemannian geometry: Harmonic maps into Euclidean Buildings
Presenter
October 23, 2024
Keywords:
- mean curvature flow
- Ricci flow
- fully nonlinear flows
- general relativity
- mass
- Ricci curvature
- scalar curvature
- sectional curvature
- symmetry
- Riemannian geometry
- groups actions
- minimal surfaces
- stability
MSC:
- 35-XX - Partial differential equations
- 49-XX - Calculus of variations and optimal control
- optimization
- 53-XX - Differential geometry
- 58-XX - Global analysis
- analysis on manifolds
- 83-XX - Relativity and gravitational theory
Abstract
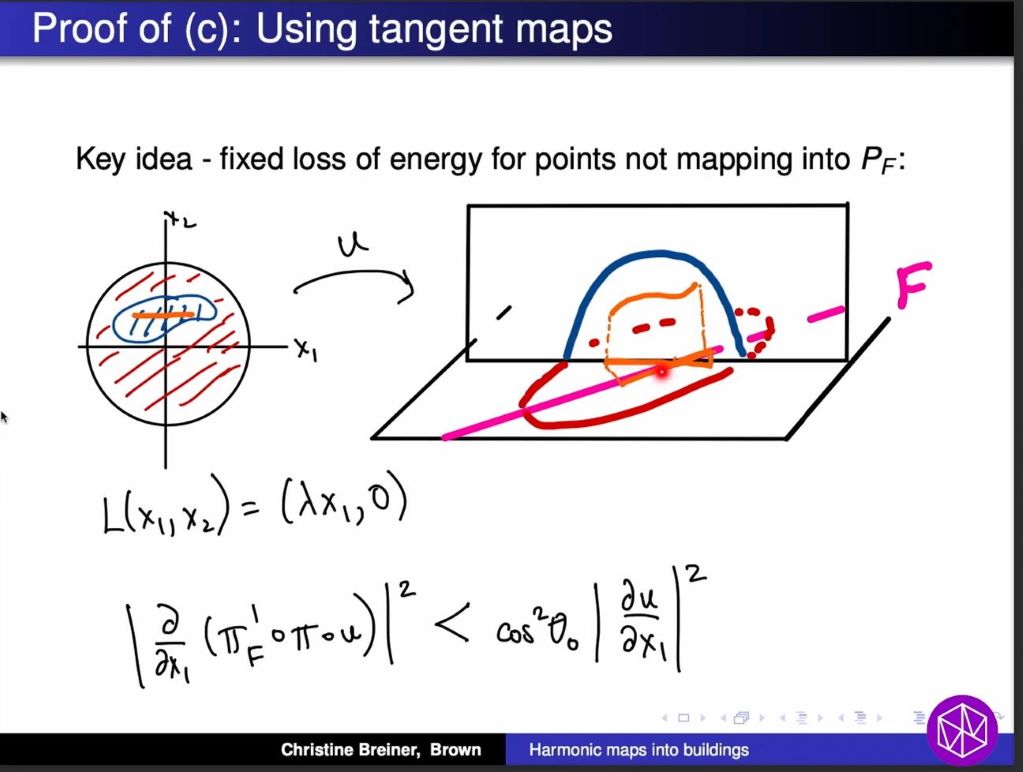
We describe a regularity result for equivariant harmonic maps from the universal cover of a Riemannian manifold into a (not necessarily locally finite) Euclidean building. As an application we prove non-Archimedean superrigidity for rank 1 symmetric spaces. This result extends the work of Gromov-Schoen, who proved p-adic superrigidity by considering locally finite targets. This work is joint with B. Dees and C. Mese.