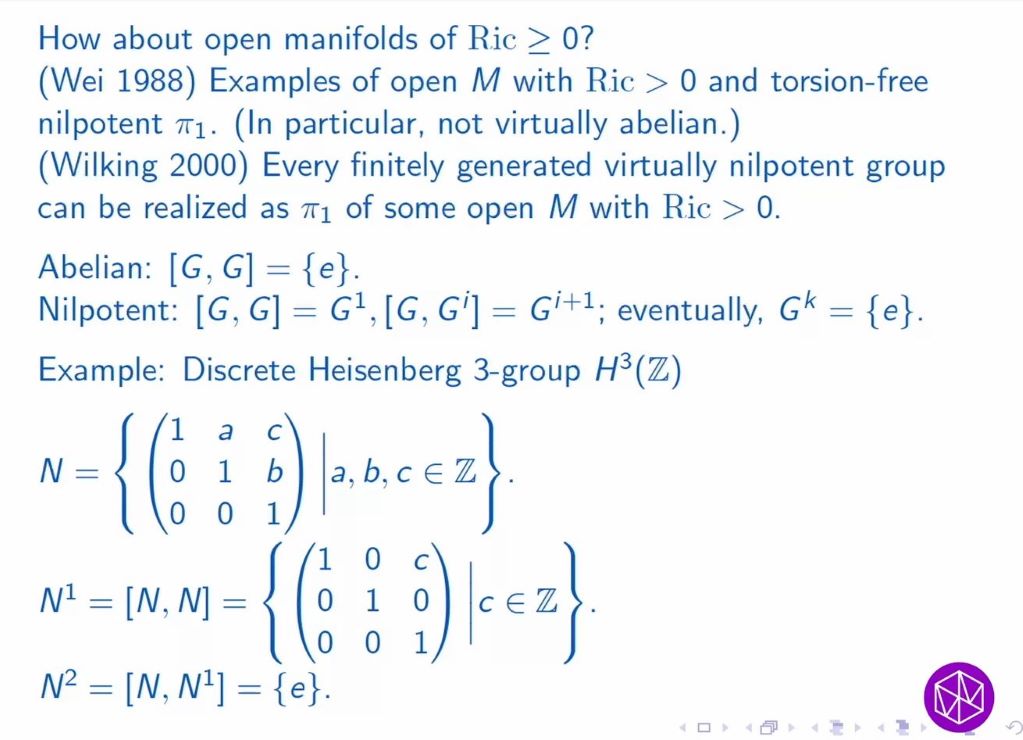
The interplay between geometry and topology is always one of the central topics in Riemannian geometry. For open (noncompact and complete) manifolds with nonnegative Ricci curvature, it is known that the fundamental groups could be torsion-free nilpotent. This is distinct from open manifolds with nonnegative sectional curvature, whose fundamental groups are virtually abelian. This talk will cover how the virtual nilpotency/abelianness of the fundamental group is related to various geometric quantities and equivariant asymptotic geometry.