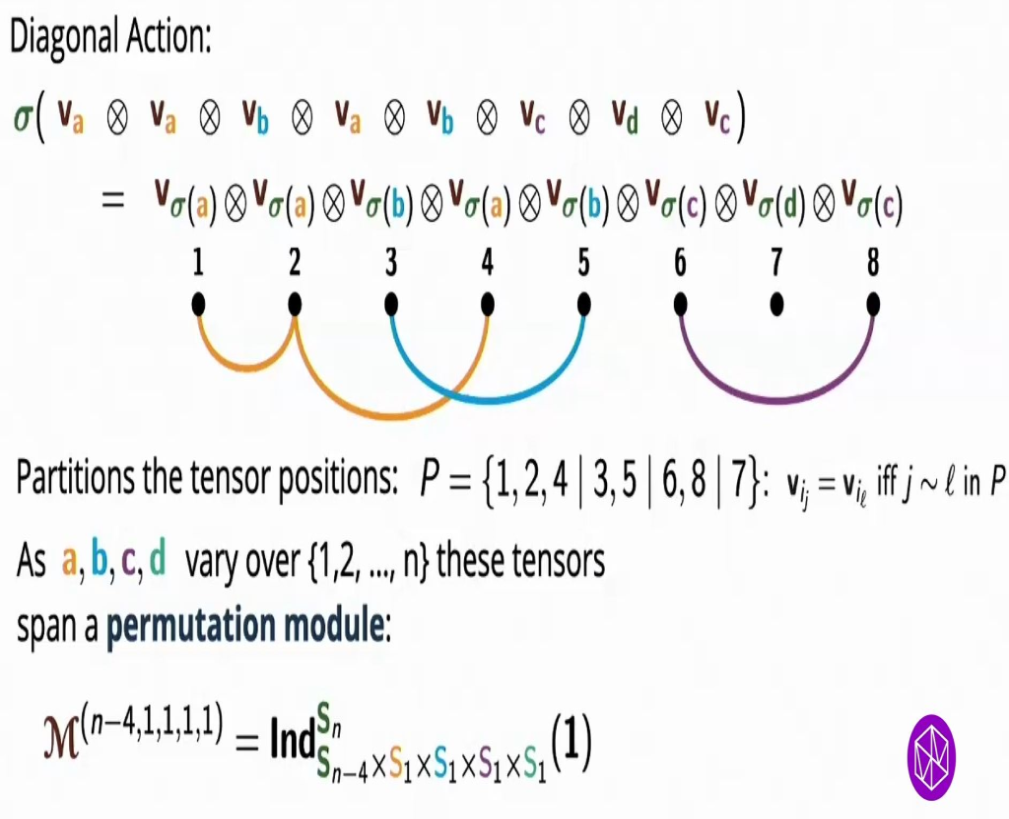
Great Permutations is a coming-of-age tale that charts the progress of the permutation representation of the symmetric group as it navigates its way through a world of tensor products. This is simultaneously a story about the multiplicity of simple modules and the dimension of centralizer modules. Throughout, various shadowy intrigues such as walks on graphs, set-partition diagrams, and even classical invariant theory enliven the story. As a sequel to the plenary talk, A Tale of Two Groups, given by Georgia Benkart at the 1994 Joint Mathematics Meetings, Great Permutations is a feel-good tale of redemption through Schur-Weyl duality that is apt to bolster your faith in representation theory.