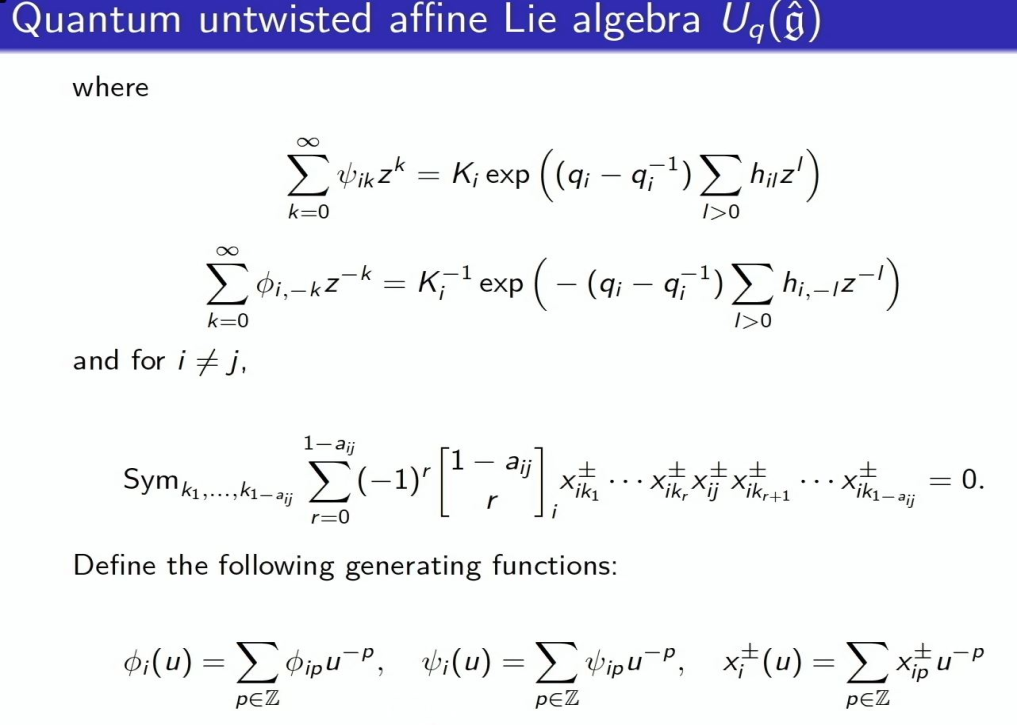
We consider reduced imaginary Verma modules for the untwisted quantum affine algebras $U_q(\hat{\g})$ and define a crystal-like base which we call imaginary crystal base using the Kashiwara algebra $\mathcal K_q$ constructed in earlier work by Ben Cox and two of the authors. We prove the existence of the imaginary crystal base for any object in a suitable category $\mc{O}^q_{red,im}$ containing the reduced imaginary Verma modules for $U_q(\hat{\g})$.