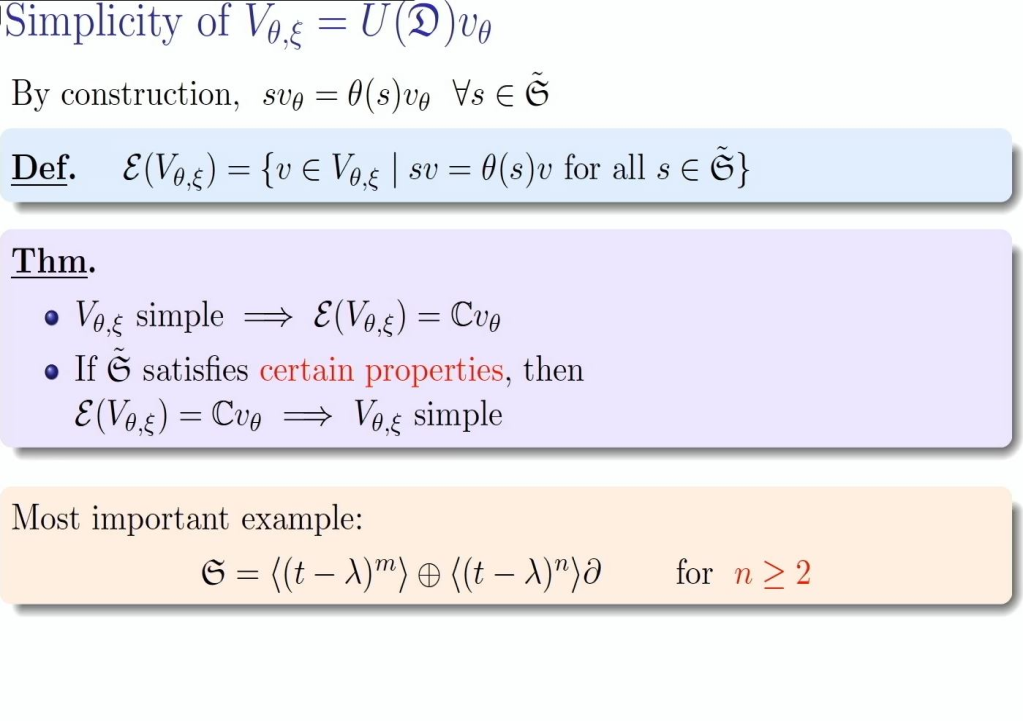
We will consider representations of the Lie algebra of differential operators of order at most one on the algebra of complex Laurent polynomials. This Lie algebra contains a natural family of large subalgebras that arise from certain pairs of Laurent polynomials. We will examine representations induced from these subalgebras, and we will see that many are in fact irreducible representations. Many of the key ideas follow from some general results concerning tensor products as well as from the fact that these subalgebras have finite codimension.