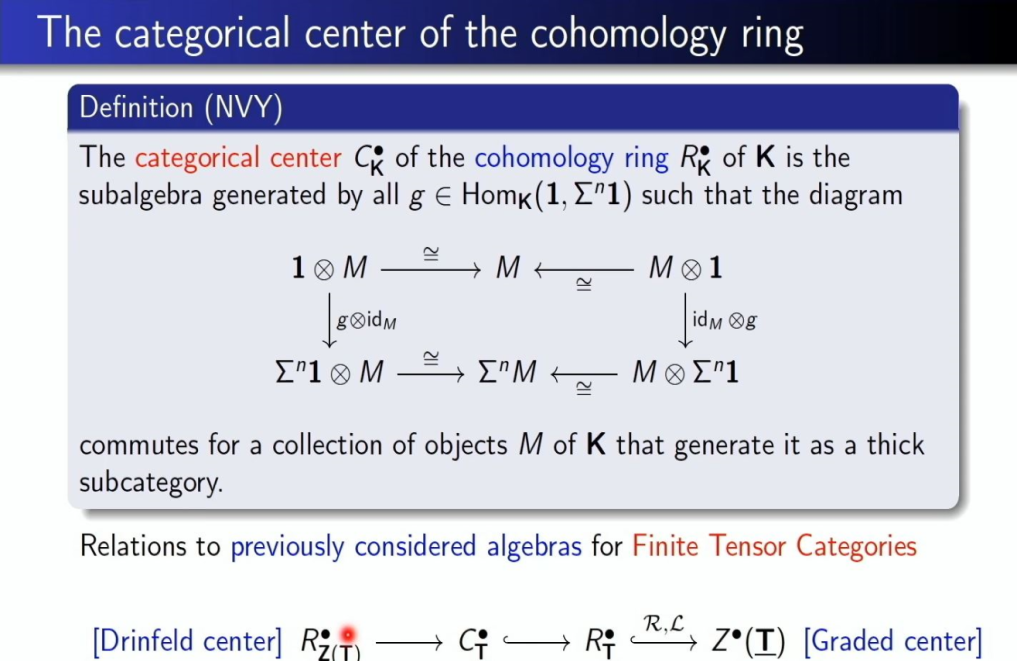
Many settings in representation theory, algebraic geometry and mathematical physics lead to monoidal triangulated categories which are generally nonsymmetric. We will present a study of their noncommutative Balmer spectra, which includes a classification of all thick ideals and a generalized Carlson's connectedness theorem. The cohomological and Balmer support maps will be linked via a notion of categorical center of the cohomology ring of a monoidal triangulated category. This is a joint work with Dan Nakano and Kent Vashaw.