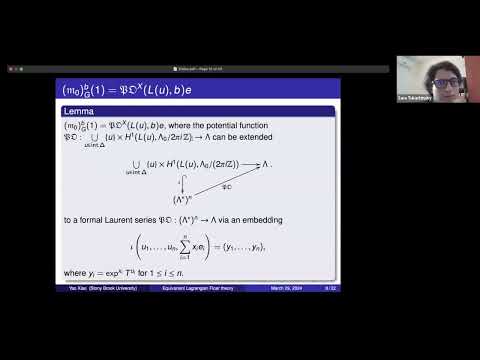
We introduce an equivariant Lagrangian Floer theory on compact symplectic toric manifolds. We define a spectral sequence to compute the equivariant Floer cohomology. We show that the set of pairs $(L,b)$, each consisting of a Lagrangian torus fiber and a weak bounding cochain, that have non-zero equivariant Lagrangian Floer cohomology forms a rigid analytic space (over the non-Archimedean Novikov field). We prove that the dimension of such a rigid analytic space is equal to that of the acting group in certain cases. We will discuss some examples.