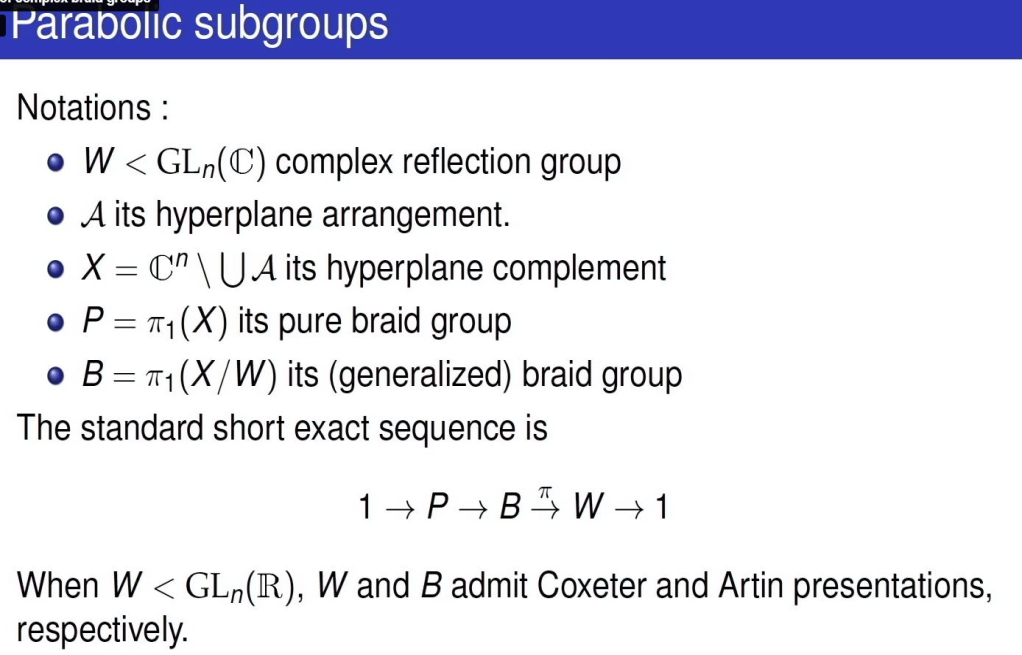
Hot Topics: Artin Groups and Arrangements - Topology, Geometry, and Combinatorics: "Parabolic subgroups of complex braid groups"
Presenter
March 15, 2024
Keywords:
- Artin groups
- braid groups
- Coxeter groups
- reflection groups
- Garside structures
- classifying spaces
- hyperplane arrangements
- subspace arrangements
- configuration spaces
- geometric group theory
- Matroids
- cohomology
MSC:
- 14N20
- 20F36
Abstract
I shall present a general definition of what should be considered a parabolic subgroup for the generalized braid group associated to a complex reflection group, and present a series of remarkable properties of these subgroups. These properties generalize the ones obtained earlier by Cumplido, Gebhardt, González-Meneses and Wiest for real groups, with a combinatorial definition of parabolic subgroups attached to a specific (Artin) presentation. First of all, intersection of parabolic subgroups are parabolic subgroups; this implies that they form a lattice of subgroups. Secondly, they are also the vertices of a graph on which the generalized braid group acts faithfully (modulo center), and which generalizes the curve graph for the usual braid group on n strands. As a consequence, this graph is conjectured to be hyperbolic. This is joint work with J. González-Meneses (Sevilla, Spain) for the most part -- only one reflection group remained untractable using our methods, a difficulty which already appeared in the proof of the K(\pi,1) conjecture for these groups. This last case has been settled recently by my student O. Garnier.