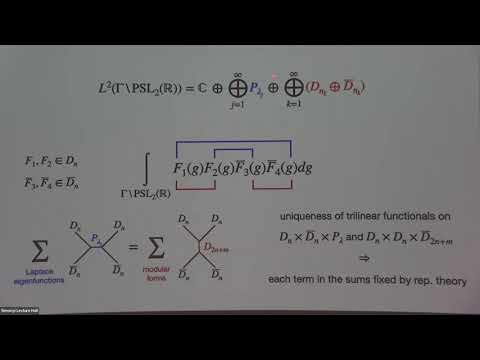
I will discuss infinite-dimensional linear programs producing bounds on the spectral gap in various settings. This includes new bounds on the spectral gap of hyperbolic manifolds as well as the Cohn+Elkies bound on the density of sphere packings. The bounds allow us to essentially determine the complete set of spectral gaps achieved by hyperbolic 2-orbifolds. The linear programs involved have been the subject of intense study by mathematical physicists in the context of the conformal bootstrap.    I will review the method of analytic extremal functionals, introduced by the speaker to prove sharp bounds in the conformal bootstrap. When used within the Cohn+Elkies linear program, this method reproduces the groundbreaking solution of Viazovska et al of the sphere packing problem in dimensions 8 and 24, as well as the interpolation basis used in the proof of universal optimality of the E8 and Leech lattice. The connections covered in this talk offer a broader framework for studying optimality in infinite-dimensional linear programs.