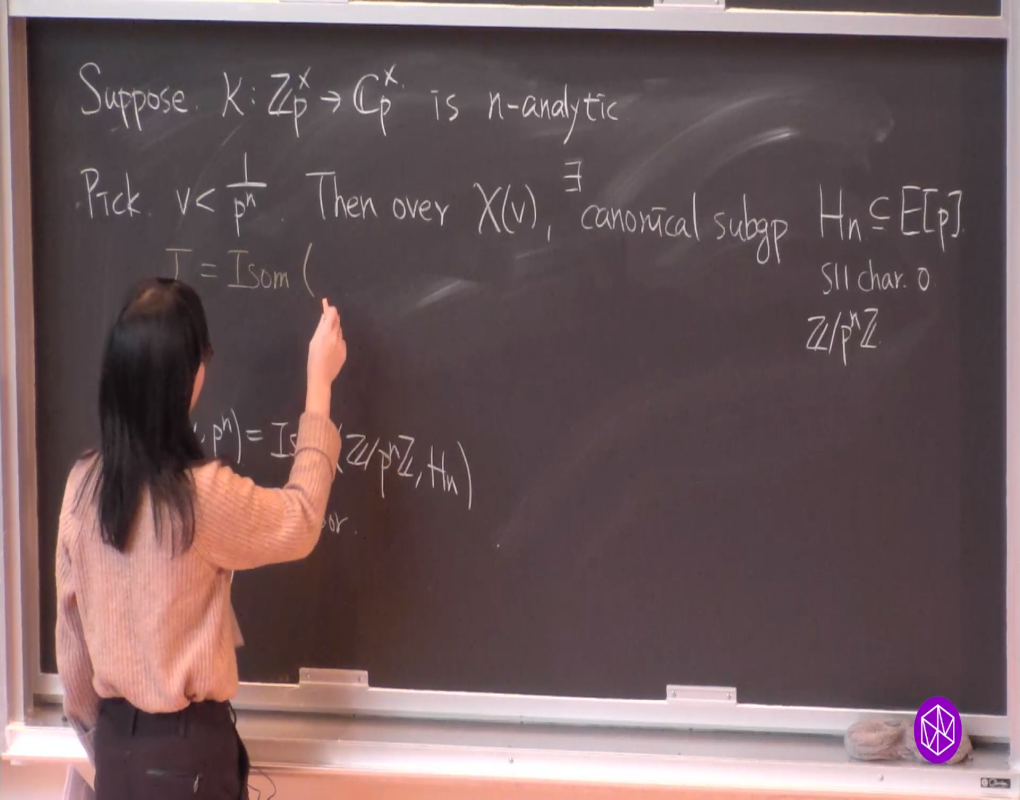It is often useful to regard modular forms as in the larger space of p-adic overconvergent modular forms, so that p-adic analytic techniques can be used to study them. The geometric interpretation of this is an eigenvariety, which is a rigid analytic space parametrizing finite-slope overconvergent Hecke eigenforms. For Hilbert modular forms, Andreatta-Iovita-Pilloni constructed p-adic families of modular sheaves as well as the eigenvariety. Moreover, for Hilbert modular forms, it makes sense to consider an intermediate notion - the partially classical overconvergent forms. I will talk about the construction of the eigenvariety in this scenario following the approach of AIP. As an application, it can be proved that the Galois representation associated to a partially classical Hilbert Hecke eigenform is partially de Rham.