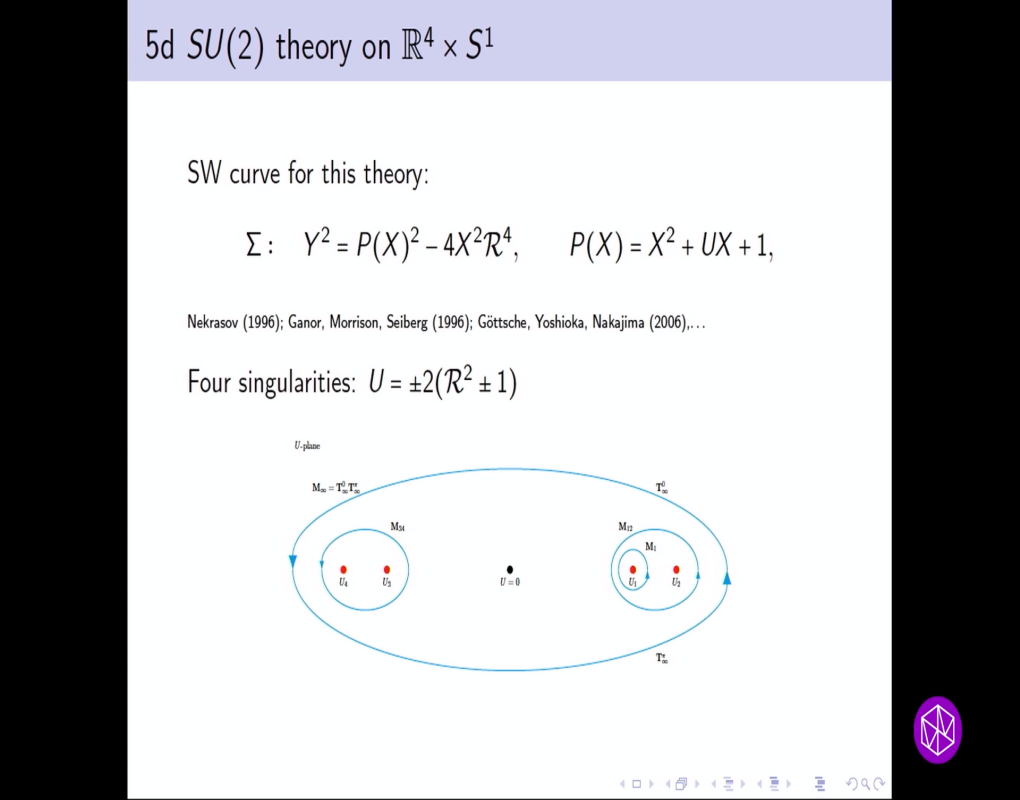
I will discuss 5-dimensional N=1 super Yang-Mills compactified on X times S^1, with X a smooth, compact, oriented 4-manifold. After a partial topological twist along X, the theory is locally independent of the metric on X, while it does depend on the radius R of S^1. The coefficients of the R-expansion of the path integral correspond to the index of a Dirac operator on moduli spaces of instantons and monopoles, or more generally K-theoretic Donaldson invariants. I will evaluate path integrals using two methods: 1) the quantum mechanics of the theory reduced to S^1 and 2) the low energy effective theory reduced to X. Both methods reproduce the same wall-crossing formula for 4-manifolds with b_2^+=1. I will also discuss the evaluation of path integrals for 4-manifolds with b_2^+>1 using method 2. Our results agree with those for algebraic surfaces by Gottsche, Nakajima and Yoshioka (2006) and Gottsche, Kool and Williams (2021). This talk is based on work in progress with H. Kim, G. Moore, R. Tao and X. Zhang.