The Teichmüller space of a surface S is the space of marked hyperbolic structure on S, up to equivalence. By considering the holonomy representation of such structures, the Teichmüller space can also be seen as a connected component of (conjugacy classes of) representations from the fundamental group of S into PSL(2,R), consisting entirely of discrete and faithful representations. Generalizing this point of view, Higher Teichmüller Theory studies connected components of (conjugacy classes of) representations from the fundamental group of S into more general semisimple Lie groups which consist entirely of discrete and faithful representations. We will give a survey of some aspects of Higher Teichmüller Theory, and will make links with the recent notion of Anosov representation. Time permitting, we will conclude by focusing on two separate questions: Do these representations correspond to deformation of geometric structures? 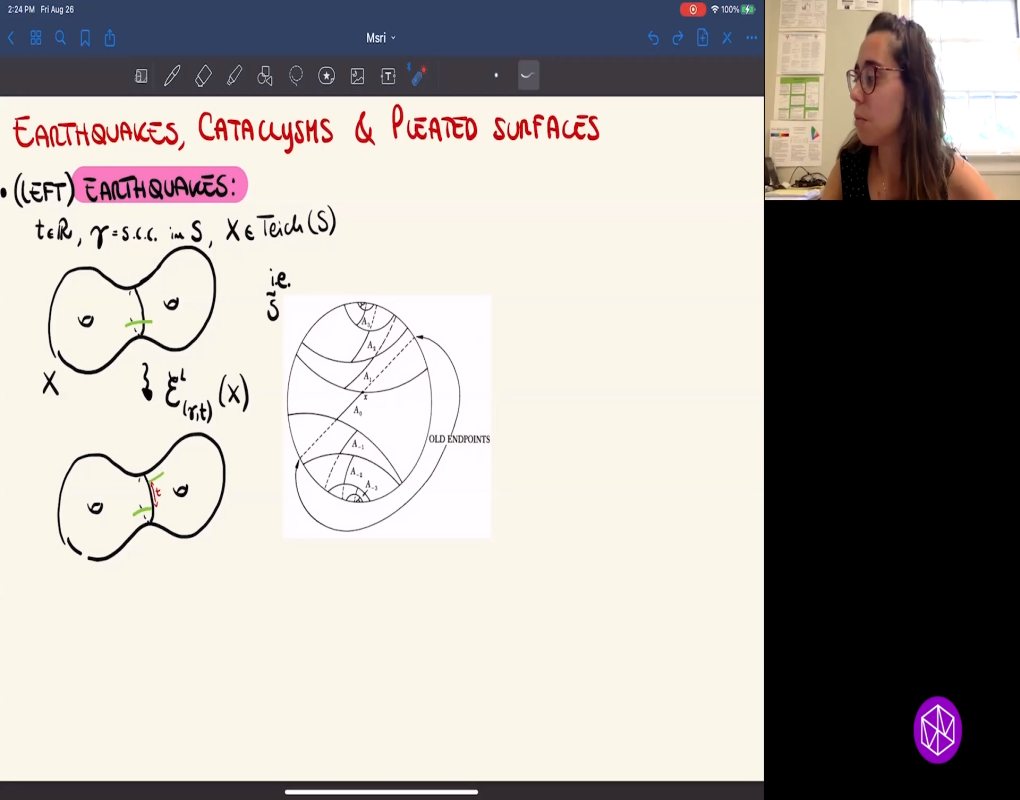Can we generalize the important notion of pleated surfaces to higher rank Lie groups like PSL(d, C)? The answer to question 1 is joint work with Alessandrini, Tholozan and Wienhard, while the answer to question 2 is joint work with Martone, Mazzoli and Zhang.