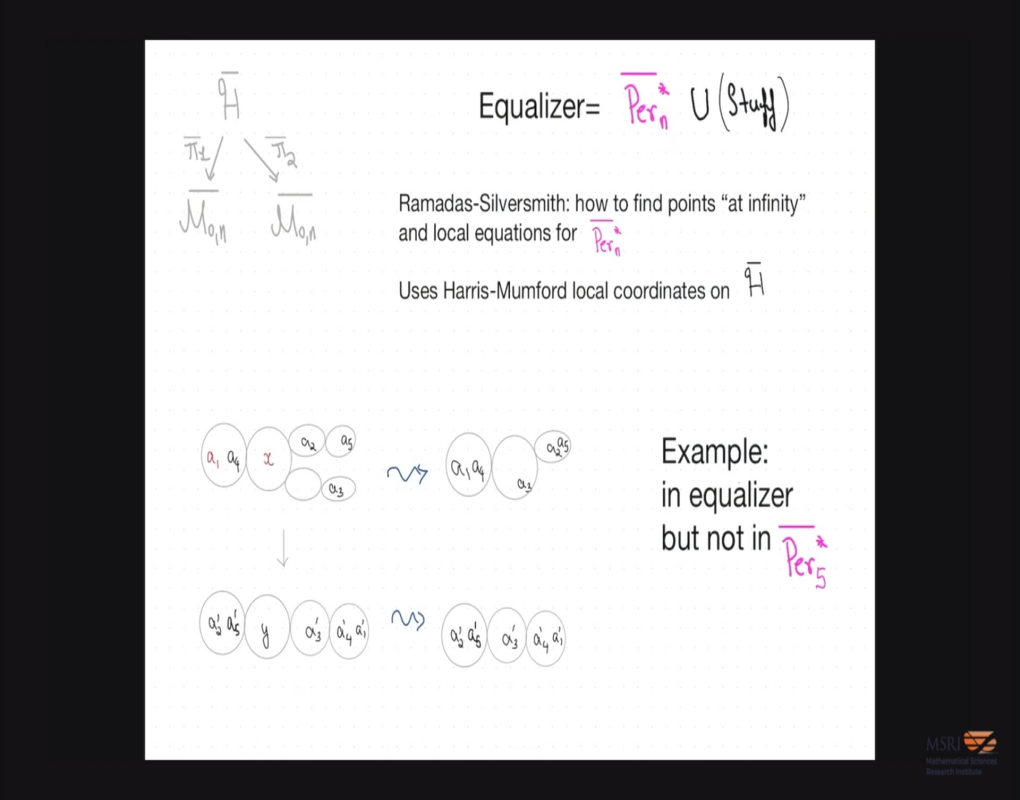
Per_n is a punctured (nodal) Riemann surface parametrizing quadratic rational maps with an n-periodic critical point; its irreducibility over C is an open question. The Gleason polynomial G_n is the polynomial whose roots are {c such that 0 is n-periodic under z^2+c}; its irreducibility over Q is an open question. I will discuss very recent work-in-progress, finding a smooth Q-rational point “at infinity” on Per_n, and using this to conclude that if G_n is irreducible over Q, then Per_n is irreducible over C. This talk will also include joint work with Rob Silversmith.