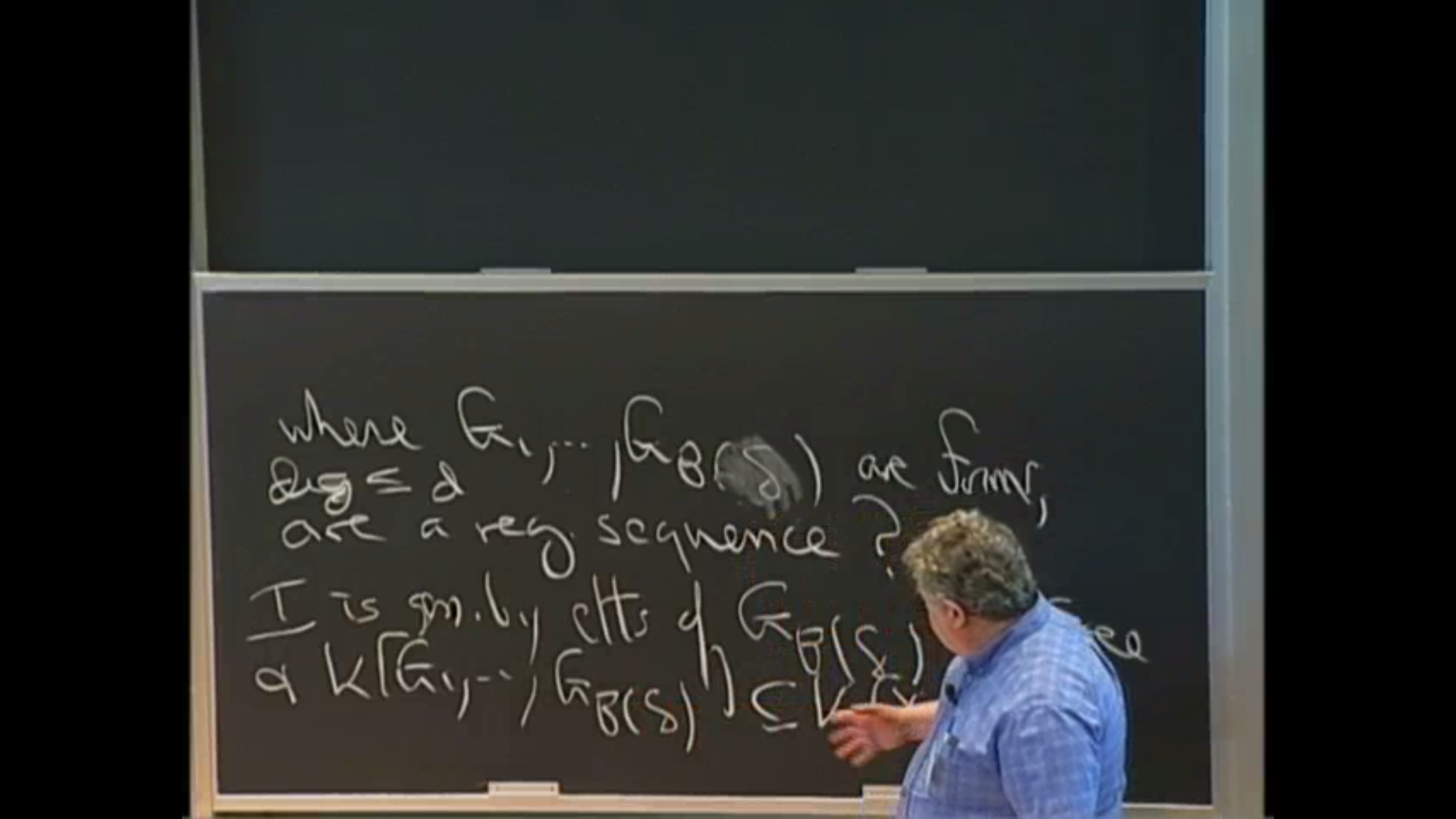The talk discusses joint work with Tigran Ananyan. Let R be a polynomial ring over an algebraically closed field K in N variables. One main result is that there is a function A(n) such that if an ideal I generated by n forms of degree at most 3 has no homogeneous minimal generator that is contained in an ideal generated by A(n) forms of lower degree, then the quotient by the ideal is a UFD. This result requires characteristic not 2,3 if cubics occur but no restriction if the forms are of degree at most 2. From this one can show that there is a function B(n) such that algebra generated by the forms is contained in similar K-algebra generated by a regular sequence consisting of at most B(n) forms. The functions A, B do not depend on N, nor on the field K. The authors earlier gave a bound for B(n) for quadrics. The new bound is much smaller. The results for cubics are new. The existence of B immediately implies an affirmative answer to a question of M. Stillman about bounding the projective dimension of R/I independent of N.