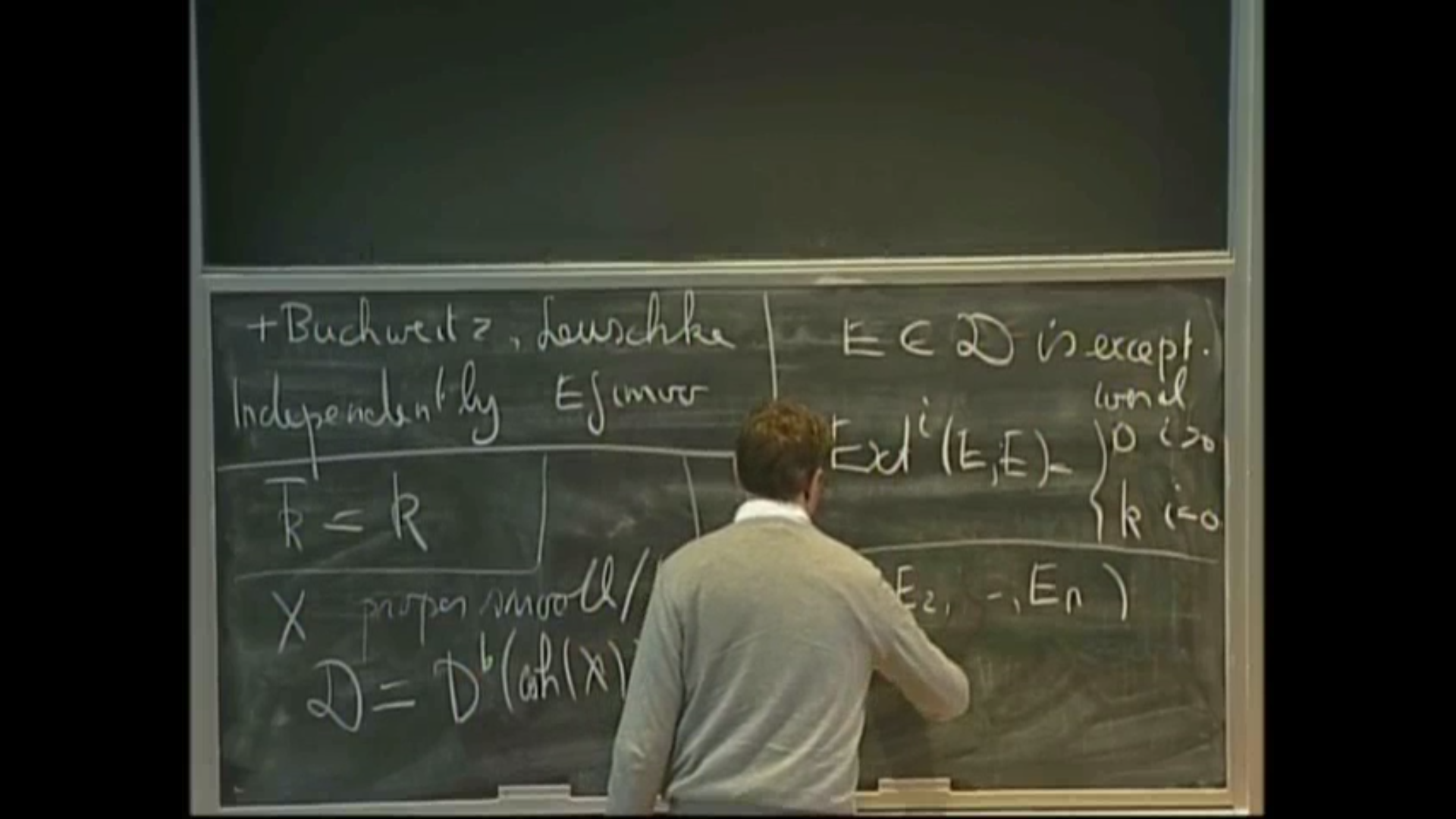Derived categories of Grassmannians in arbitrary characteristic
Presenter
April 10, 2013
Keywords:
- derived categories
- Grassmannians
- Schur algebras
- noncommutative algebra
- noncommutative algebraic geometry
- representation theory
- D-module
- deformation quantization
MSC:
- 16E35
- 16Exx
- 14M15
- 14F10
- 14Fxx
- 14-xx
- 16Gxx
- 81-xx
- 81Rxx
- 81R50
- 81R60
- 81Sxx
- 81S10
Abstract
We discuss joint work with Ragnar Buchweitz and Graham Leuschke on the derived category of Grassmannians in arbitrary characteristic. Generalizing Kapranov's well-known characteristic zero results we construct dual exceptional collections on them (which are however not strong) as well as a tilting bundle. We show that this tilting bundle has a quasi-hereditary endomorphism ring and we identify the standard, costandard, projective and simple modules of the latter. The basic technical tool is a semi-orthogonal decomposition of the derived category whose parts are given by generalized Schur algebras.