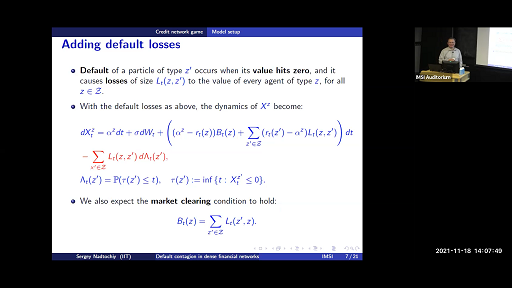
I will describe a dynamic mean-field game on a dense heterogeneous directed network (graphon) which models the propagation of default losses in an interconnected financial system. In this game, each agent (a node of the network) chooses her outgoing (lending) connections strategically, in order to maximize the expected value of her future cash flow. A default of any agent shifts the values of her creditors downward. It is well known that without the strategic behavior such systems exhibit blow-ups — when a non-zero fraction of the population default at the same time. Remarkably, in equilibrium, the agents choose to adjust their strategies in order to minimize their exposure to the most vulnerable counter-parties, and the blow-ups never occur. On the mathematical side, the main challenge of this work is in constructing a mean-field equilibrium (which is also the “minimal” equilibrium) in this game, which is split into a dynamic and a static parts. The static part corresponds to constructing an equilibrium in a decentralized network-flow game, which is interesting in its own right. In particular, our solution to the static problem allows one to determine the distribution of a capital injection across the financial institutions so that the overall flow of capital to the real economy is maximized. Based on a joint work with M. Shkolnikov.