Dynamics of Complex Henon Maps
Presenter
February 3, 2022
Keywords:
- complex Henon maps
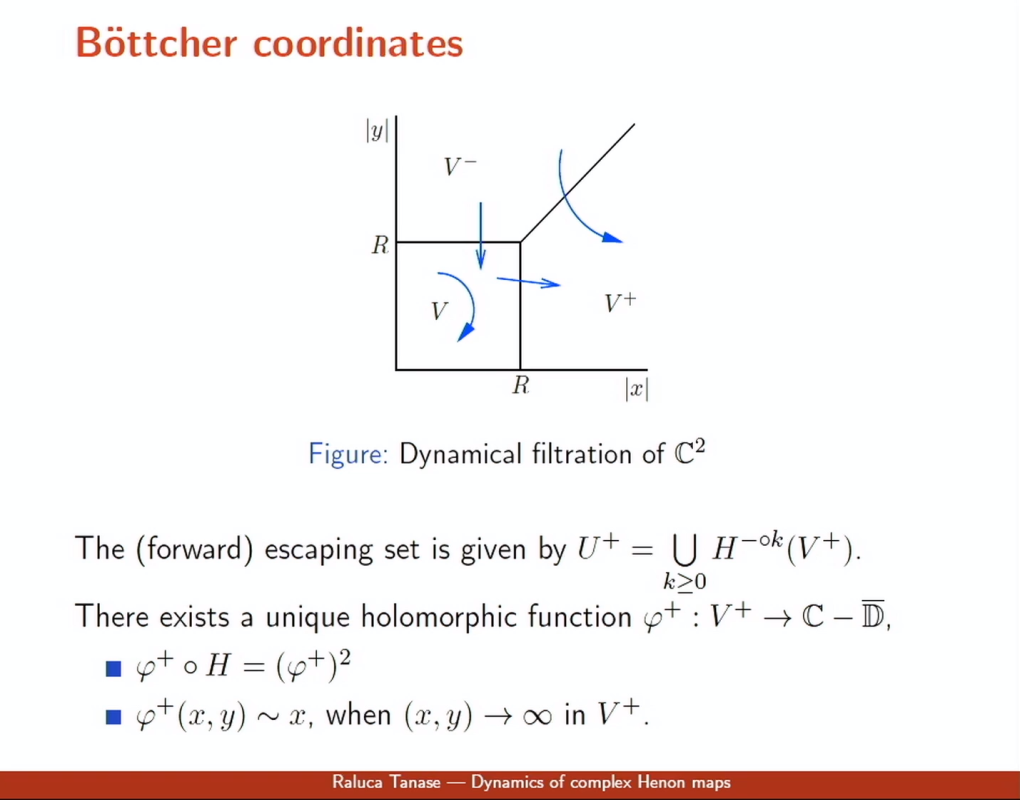
- escaping sets
- foliations
- critical locus
- higher dimensional holomorphic dynamics
MSC:
- 37F75
- 37F15
- 32H50
- 32A10
Abstract
In this talk we discuss the dynamics of the complex Henon map, a prototype of a 2D dynamical system exhibiting stretching, folding, and chaos. We introduce several invariant objects and discuss their dynamical properties, emphasizing important advances in the field. In particular, we talk about the critical locus, an exotic and mysterious set associated with the Henon map. In 1D, critical points play an essential role in the dynamics of polynomial Julia sets. In 2D, a Henon map does not have critical points in the usual sense, but it has a non-empty critical locus (i.e. the set of tangencies between the foliations of the forward and backward escaping sets), which we analyze in a broader, non-perturbative context. This is based on joint work with Tanya Firsova and Remus Radu.