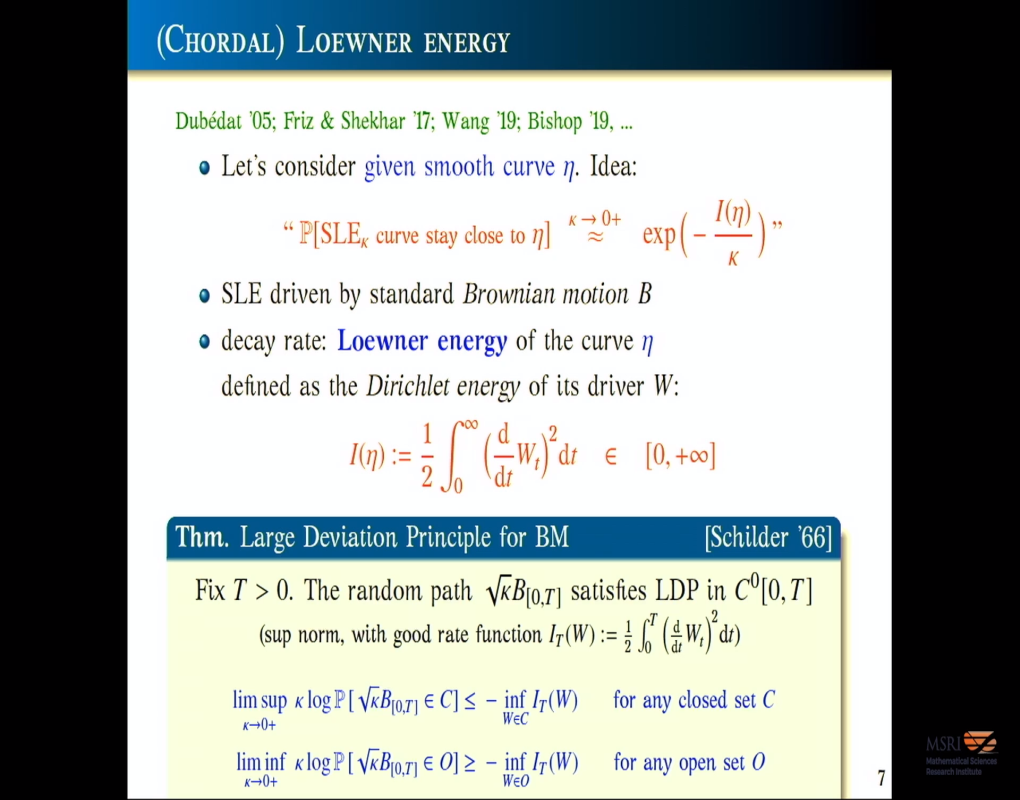
When studying large deviations (LDP) of Schramm-Loewner evolution (SLE) curves, we recently introduced a ''Loewner potential'' that describes the rate function for the LDP. This object turned out to have several intrinsic, and perhaps surprising, connections to various fields. For instance, it has a simple expression in terms of zeta-regularized determinants of Laplace-Beltrami operators. On the other hand, minima of the Loewner potential solve a nonlinear first order PDE that arises in a semiclassical limit of certain correlation functions in conformal field theory, arguably also related to isomonodromic systems. Finally, and perhaps most interestingly, the Loewner potential minimizers classify rational functions with real critical points, thereby providing a novel proof for a version of the now well-known Shapiro-Shapiro conjecture in real enumerative geometry. This talk is based on joint work with Yilin Wang (MIT) - see also Yilin's talk in the next workshop!