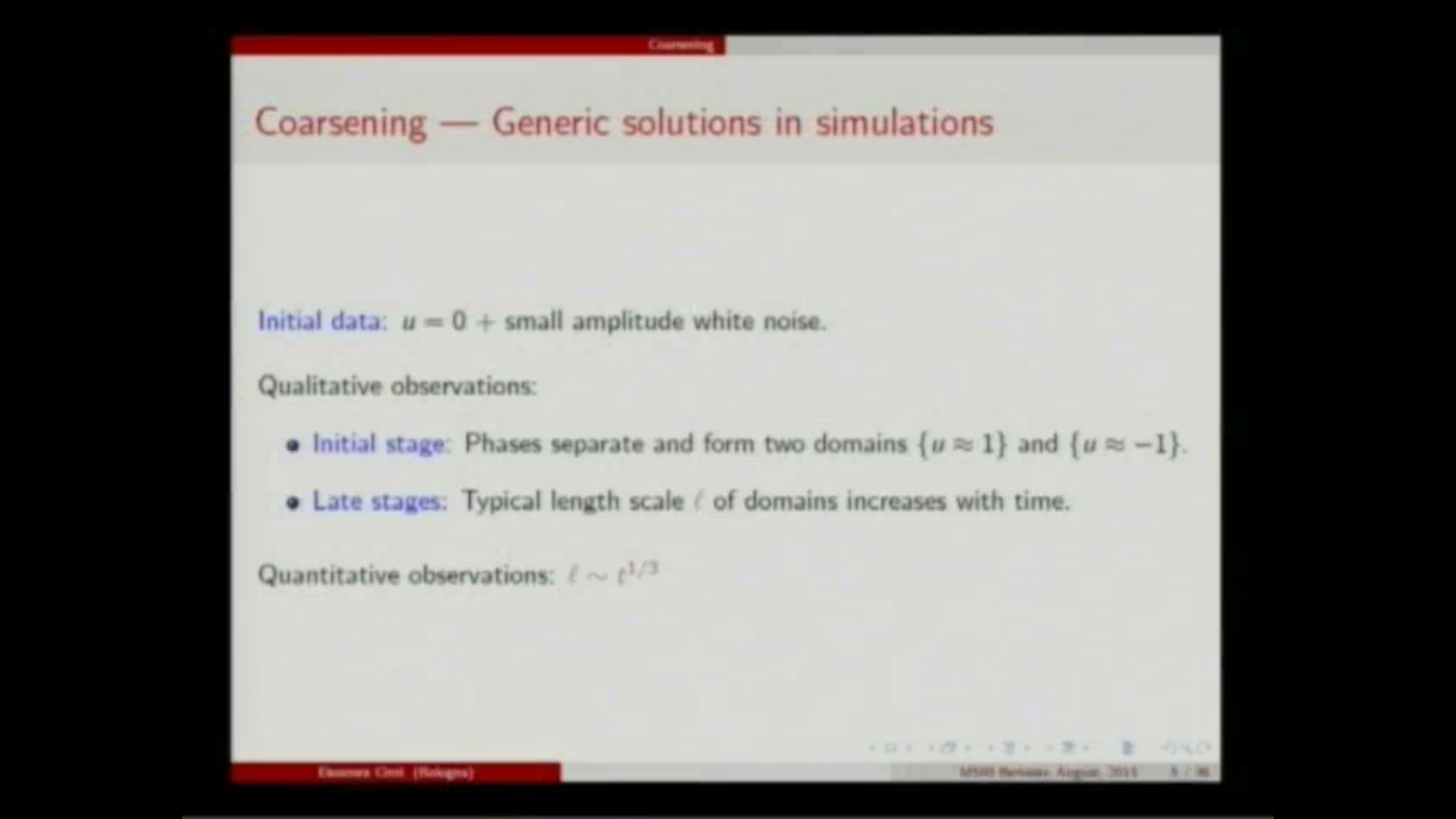
In this talk I will present some interpolation inequalities which arise in the study of pattern formation in physics. In many physical problems described by a variational model (such as domain branching in ferromagnets, superconductors, twin branching in shape memory alloys), the energy is given by the competition of two main terms: an interfacial energy (described by a BV-norm) and a transport term (described by a negative norm or a Wasserstein distance). In order to establish a rigorous lower bound for the energy of minimizing configurations, one needs suitable interpolation inequalities. I will describe the connection between these interpolation estimates and the physical problem, and I will sketch the proof of some of these estimates. This is a joint work with Felix Otto.