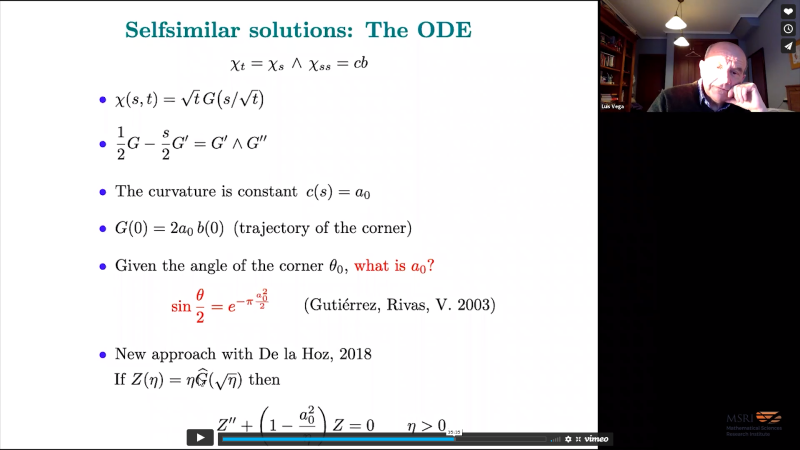
Riemann's non-differentiable function and the binormal curvature flow
Presenter
April 15, 2021
Keywords:
- RIEMANN’S NON-DIFFERENTIABLE FUNCTION
- binormal flow
MSC:
- 35Qxx
- 35J05
- 35J10
- 35K05
- 35L05
Abstract
In a joint work with Valeria Banica, we make a connection between a famous analytical object introduced in the 1860s by Riemann, as well as some variants of it, and a nonlinear geometric PDE, the binormal curvature flow. As a consequence this analytical object has a non-obvious non- linear geometric interpretation. We recall that the binormal flow is a standard model for the evolution of vortex filaments. We prove the existence of solutions of the binormal flow with smooth trajectories that are as close as desired to curves with a multifractal behavior. Finally, we show that this behavior falls within the multifractal formalism of Frisch and Parisi, which is conjectured to govern turbulent fluids. If time permits, I will also mention some recent results on intermittency in the context of the linear Schrödinger equation.