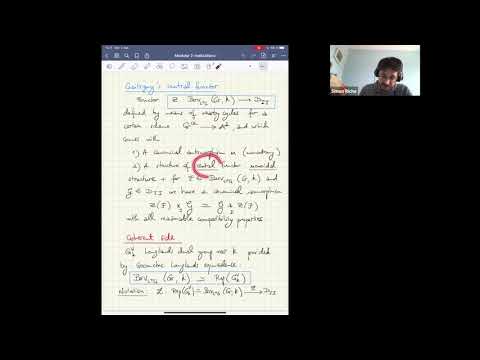
I will report on a project joint with Roman Bezrukavnikov (and partly with Laura Rider) aiming at constructing a variant for positive-characteristic coefficients of the equivalence constructed by Bezrukavnikov in "On two geometric realizations of an affine Hecke algebra". In a first paper we have obtained a description of the "regular quotient" of the category of Iwahori-equivariant perverse sheaves on the affine flag variety in terms of representations of the centralizer of a regular unipotent element in the dual group. In a second paper (in preparation) we obtain a version of this equivalence "in families" over the adjoint quotient, which allows to obtain the desired equivalence "over the regular locus". In the talk I will explain these constructions, and how we plan to use them to construct the full equivalence.