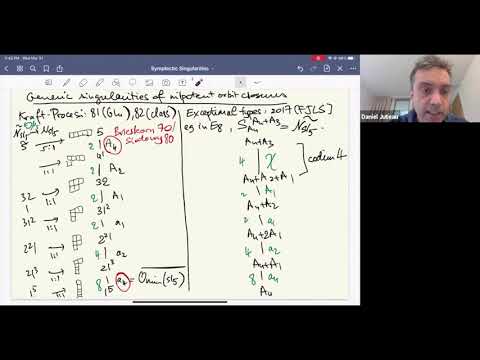
In 2000, Arnaud Beauville introduced the notion of symplectic singularities and raised the question of classifying isolated symplectic singularities with trivial local fundamental group: the latter condition is meant to avoid the numerous quotient singularities, since their classification would become intractable as the dimension increases. Actually, he asked whether there was any example beyond minimal nilpotent orbit closures in simple Lie algebras. We will describe a family of four-dimensional examples related to dihedral groups, which can be described in several ways: blow-up of quotient singularities, Calogero-Moser spaces, or covers of nilpotent slices, with Nakajima quiver varieties providing connections between the last two. The one attached to the dihedral group of order 10 appears in the nilpotent cone of type $E_8$. This is joint work with Gwyn Bellamy, Cédric Bonnafé, Baohua Fu, Paul Levy, and Eric Sommers.