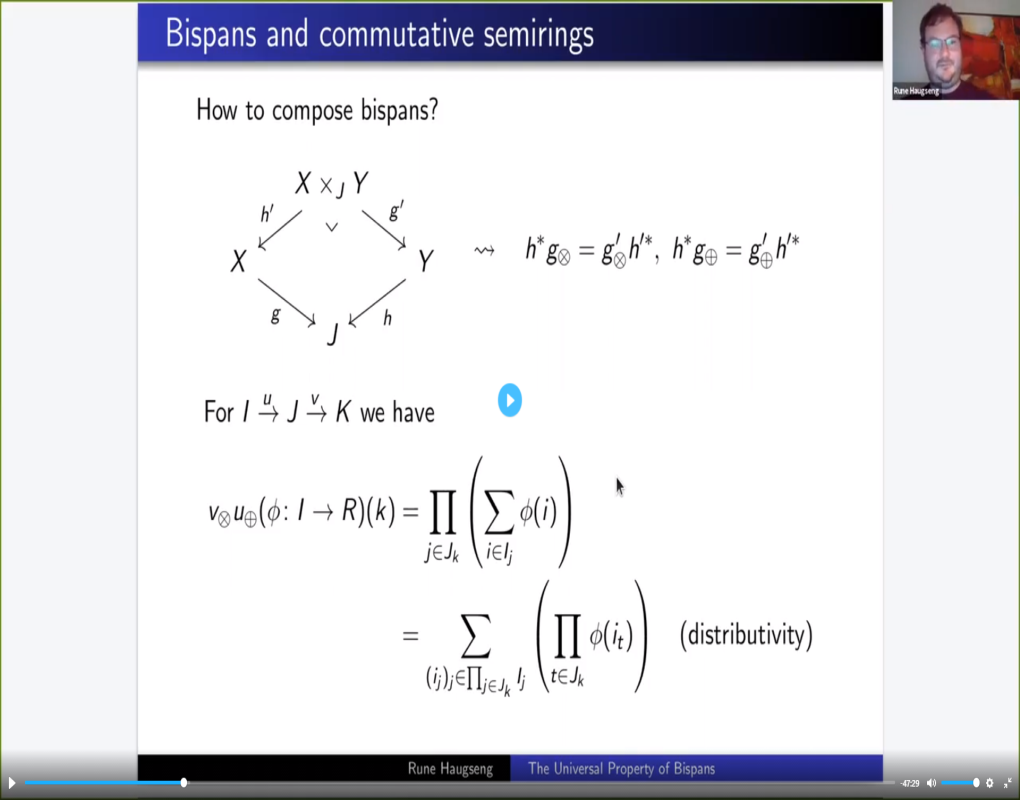
The universal property of bispans
Presenter
March 27, 2020
Keywords:
- Bispans
- semirings
- $(\infty
- 2)$-categories
MSC:
- 18D05
- 18B10
- 16Y60
- 18C10
Abstract
Commutative semirings can be described in terms of bispans of finite sets, meaning spans with an extra forward leg; if we instead take bispans in finite G-sets we get Tambara functors, which are the structure on $\pi_0$ of $G$-equivariant commutative ring spectra. Motivated by applications of the $\infty$-categorical upgrade of such descriptions to motivic and equivariant ring spectra, I will discuss the universal property of $(\infty,2)$-categories of bispans. I will focus on the simplest case of bispans in finite sets, where this gives a new construction of the semiring structure on a symmetric monoidal $\infty$-category whose tensor product commutes with coproducts. This is joint work with Elden Elmanto.