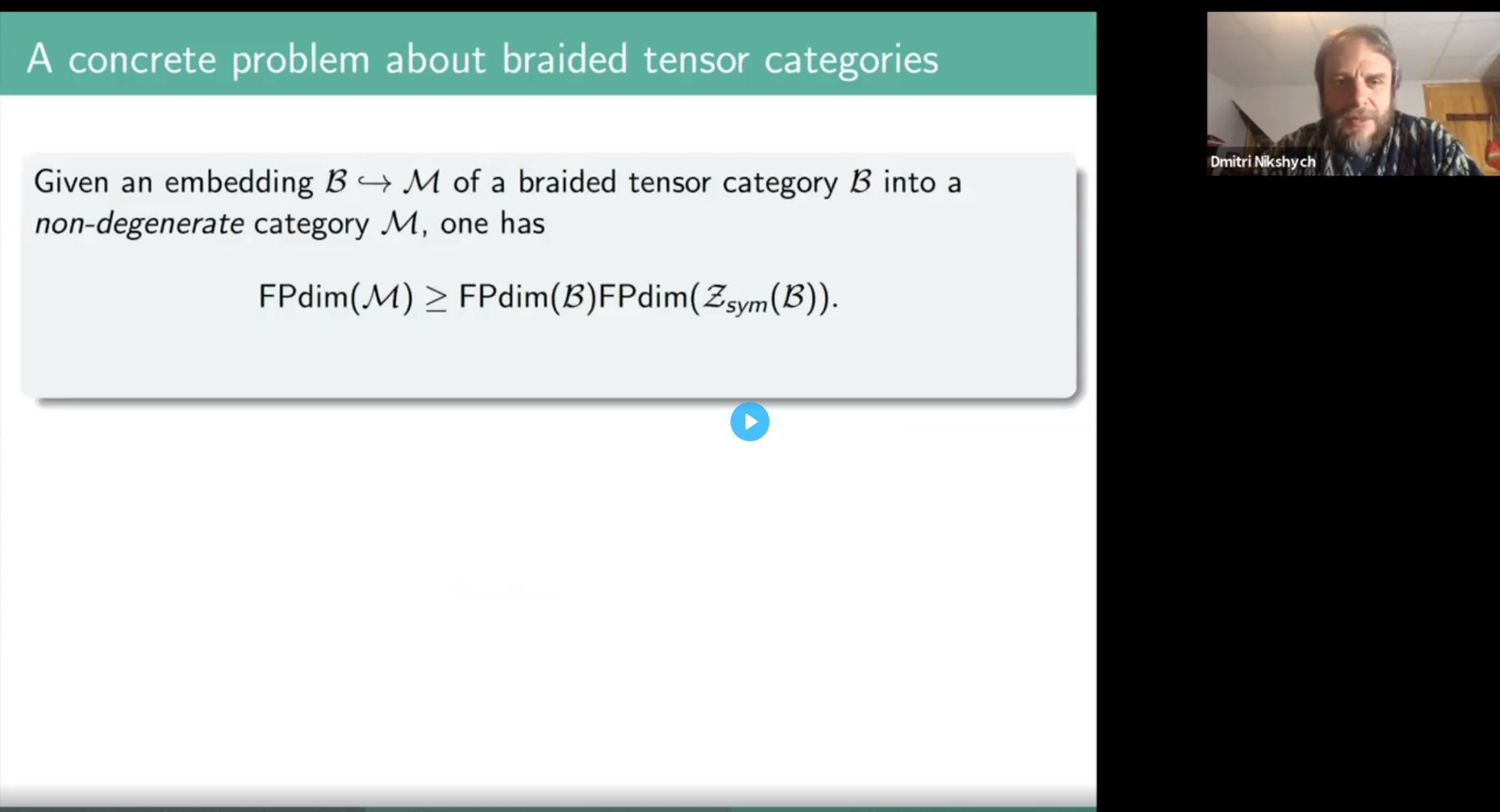
This is a report on the joint work of Alexei Davydov and the speaker. Let B be a braided tensor category. A non-degenerate braided category M containing B is called a minimal extension if the centralizer of B in M coincides with the symmetric center of B. We will discuss the existence problem for minimal extensions. When the symmetric center is pointed, this problem can be approached using the braided Picard group of B. We compute the (higher categorical) Lan-Kong-Wen group of minimal extensions of a symmetric fusion category in this case.