Morgan Weiler, Rice University: Infinite staircases of symplectic embeddings of ellipsoids into Hirzebruch surfaces Gromov nonsqueezing tells us that symplectic embeddings are governed by more complex obstructions than volume. In particular, in 2012, McDuff-Schlenk computed the embedding capacity function of the ball, whose value at a is the size of the smallest four-dimensional ball into which the ellipsoid E(1,a) symplectically embeds. They found that it contains an “infinite staircase” of piecewise-linear sections accumulating from below to the golden ratio to the fourth power. However, infinite staircases seem to be rare for more general targets. Work of Cristofaro-Gardiner-Holm-Mandini-Pires suggests that, up to scaling, there are only finitely many rational symplectic toric manifolds whose embedding capacity functions contain infinite staircases, while Usher has found infinitely many irrational polydisks with infinite staircases. Using ECH capacities in conjunction with the methods of McDuff-Schlenk, we will explain how we have found several infinite families of Hirzebruch surfaces whose embedding capacity functions we expect to contain an infinite staircase. Many of these staircases are “descending” rather than “ascending." This is joint work with Maria Bertozzi, Tara Holm, Emily Maw, Dusa McDuff, Grace Mwakyoma, and Ana Rita Pires. 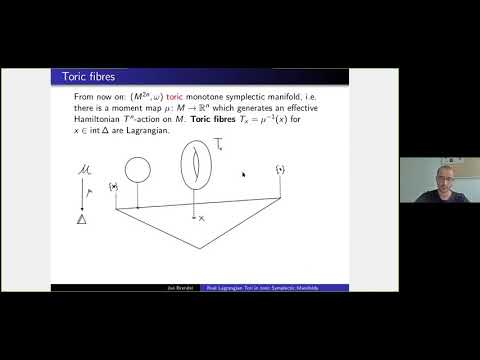Joé Brendel, University of Neuchâtel : Real Lagrangian Tori in toric symplectic manifolds In this talk we will be addressing the question whether a given Lagrangian torus in a toric monotone symplectic manifold can be realized as the fixed point set of an anti-symplectic involution (in which case it is called "real"). In the case of toric fibres, the answer depends on the geometry of the moment polytope of the ambient manifold. In the case of the Chekanov torus, the answer is always no. This can be proved using displacement energy and versal deformations. Abror Pirnapasov, Ruhr-Universität Bochum : Reeb orbits that force topological entropy A transverse link in a contact 3-manifold forces topological entropy if every Reeb flow possessing this link as a set of periodic orbits has positive topological entropy. We will explain how cylindrical contact homology on the complement of transverse links can be used to show that certain transverse links force topological entropy. As an application, we show that on every closed contact 3-manifold exists transverse knots that force topological entropy. We also generalize to the category of Reeb flows a beautiful result due to Denvir and Mackay, which says that if a Riemannian metric on the two-dimensional torus has a contractible closed geodesic then its geodesic flow has positive topological entropy. All this is joint works with Marcelo R.R. Alves, Umberto L. Hryniewicz and Pedro A.S. Salomão