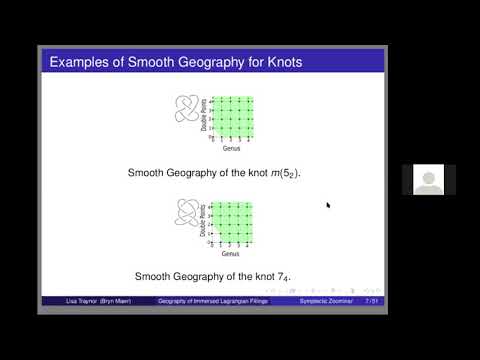
Given a smooth knot K in the 3-sphere, a classic question in knot theory is: What surfaces in the 4-ball have boundary equal to K? One can also consider immersed surfaces and ask a “geography” question: What combinations of genus and double points can be realized by surfaces with boundary equal to     K?  I will discuss symplectic analogues of these questions:  Given a Legendrian knot, what Lagrangian surfaces can it bound? What immersed Lagrangian surfaces can it bound?  These Lagrangian surfaces are commonly called Lagrangian fillings of the Legendrian knot and are more rigid than their topological counterpart.  In particular, while any smooth knot bounds an infinite number of topologically distinct surfaces, there are classical and non-classical obstructions to the existence of Lagrangian fillings of Legendrian knots.  Specifically, a polynomial associated to the Legendrian boundary through the technique of generating families can show that there is no compatible embedded Lagrangian filling.  Immersed Lagrangian fillings are more flexible, and I will describe how this polynomial associated to the Legendrian boundary forbids particular combinations of genus and double points in immersed Lagrangian fillings.  In addition, I will describe some constructions of immersed fillings that allow us to completely answer the Lagrangian geography question for some Legendrian knots.  This is joint work with Samantha Pezzimenti.