Algebraic Dynamics and the Model Theory of Difference Fields
Presenter
February 7, 2014
Keywords:
- dynamical Mordell-Lang conjecture
- dynamical Manin-Mumford conjecture
- special points
- difference fields
- orthogonality
- Zilber trichotomy
MSC:
- 03Cxx
- 37P55
- 37P50
- 37P20
- 37P35
- 37Pxx
- 37-xx
Abstract
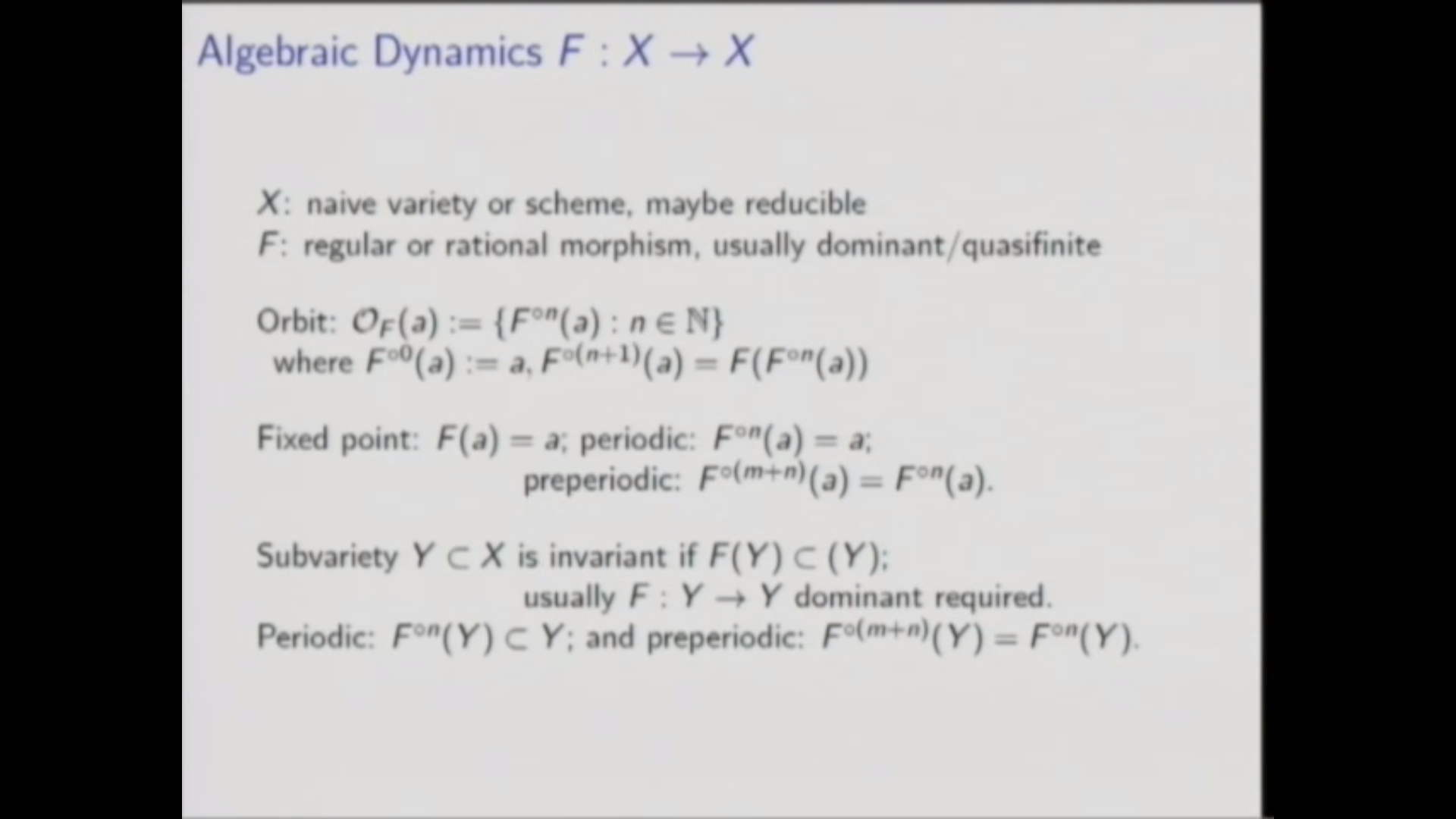
An algebraic dynamical system is a variety $X$ with a self-morphism $F : X \rightarrow X$. Difference equations are a natural tool for understanding such discrete dynamical systems, just as differential equations are natural toop for understanding continuous dymanical systems. "Model theory of difference fields" (1999) by Chatzidakis and Hrushovski is a beautiful application of geometric stability theory (one of the shiniest parts of modern model theory!) to algebraic difference equations. Several results in arithmetic dynamics have now been obtained through this model-theoretic way of thinking about difference equations. I will describe several of these, including the work on Chatzidakis and Hrushovski on descent in algebraic dynamics and my work with Scanlon on coordinate-wise polynomial dynamics.