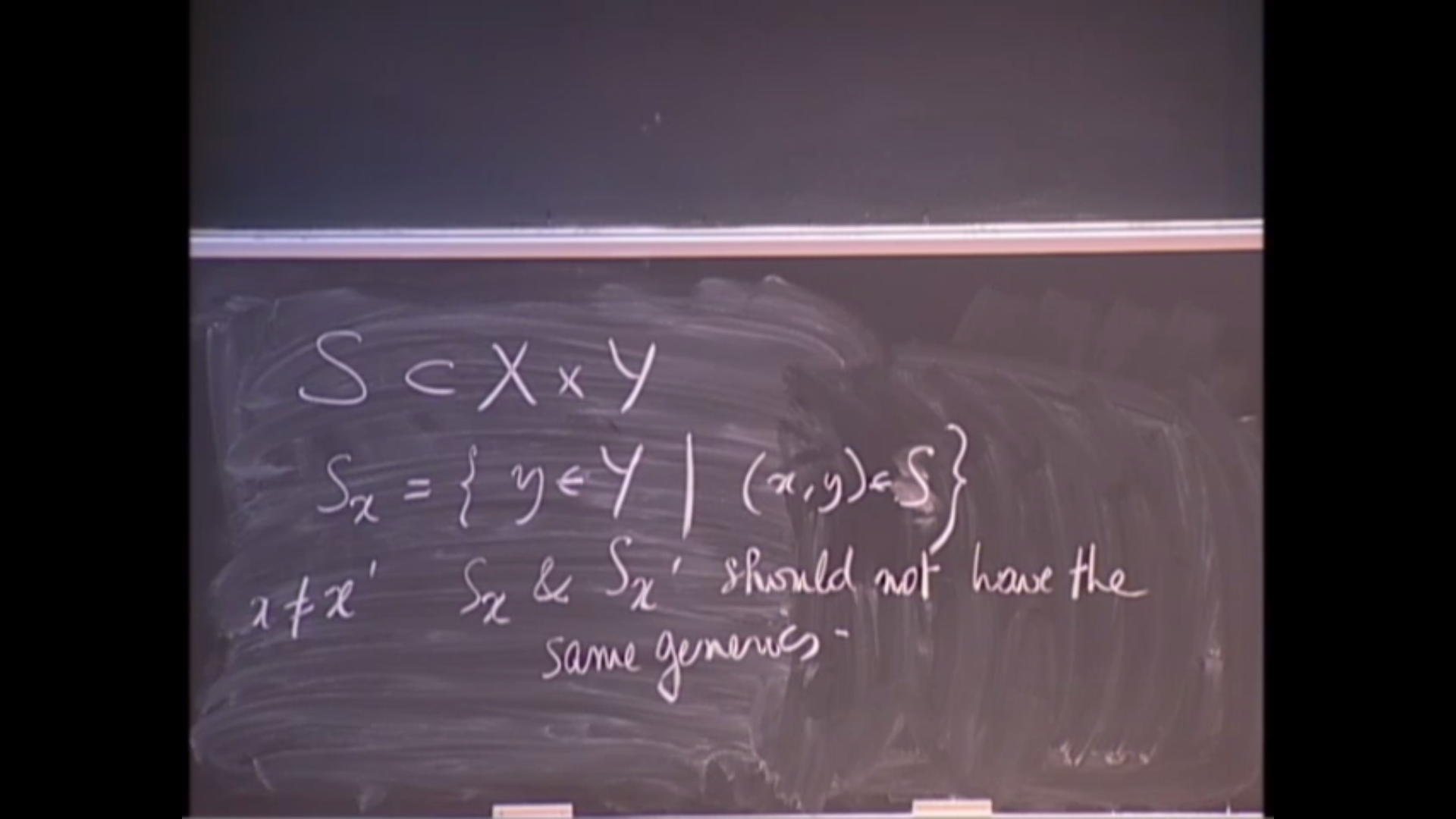Around the Canonical Base Property
Presenter
February 11, 2014
Keywords:
- finite rank
- model theory
- differential field
- Manin-Mumford conjecture
MSC:
- 03Cxx
- 03C40
- 03C60
- 03C62
Abstract
The Canonical Base Property (CBP for short) was introduced by Pillay, inspired by results in Complex geometry. It implies the dichotomy "modular/fields" for rank 1 types, and is satisfied by the two main examples of fields with operators. After giving the definition, I will discuss how one can prove it, some of its consequences, notably a descent result. I will also mention some applications to algebraic questions.